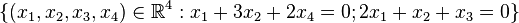Übungen 09: Unterschied zwischen den Versionen
(→Aufgabe 7) |
SelOWö (Diskussion | Beiträge) (→Aufgabe 2) |
||
| Zeile 7: | Zeile 7: | ||
a) Prüfen Sie, ob die Vektoren <math>v_1 = (4,4,4),\; v_2 = (2,4,6)</math> und <math>v_3 = (3,4,5)</math> ein Erzeugendensystem von<math> {\mathbb R}^3</math> bilden.<br /> | a) Prüfen Sie, ob die Vektoren <math>v_1 = (4,4,4),\; v_2 = (2,4,6)</math> und <math>v_3 = (3,4,5)</math> ein Erzeugendensystem von<math> {\mathbb R}^3</math> bilden.<br /> | ||
b) Untersuchen Sie, für welche <math>t \in {\mathbb R}</math> die Vektoren <math>v_1 = (1,3,4)\,, \;\, v_2 = (3,t,11)\,, \;\, v_3 = (-4,-4,0)</math> linear abhängig in <math>{\mathbb R}^3</math> sind. | b) Untersuchen Sie, für welche <math>t \in {\mathbb R}</math> die Vektoren <math>v_1 = (1,3,4)\,, \;\, v_2 = (3,t,11)\,, \;\, v_3 = (-4,-4,0)</math> linear abhängig in <math>{\mathbb R}^3</math> sind. | ||
| + | |||
| + | |||
| + | zu b) | ||
| + | ich habe raus, dass für t=50/6 die Vektoren linear unabhängig sind, das heißt für t ungleich 50/6 sind sie linear abhängig. Stimmt das? | ||
==Aufgabe 3== | ==Aufgabe 3== | ||
Version vom 3. Juli 2013, 13:26 Uhr
Inhaltsverzeichnis |
Aufgabe 1
Zeigen Sie, dass die Vektoren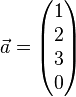 
, 
, 
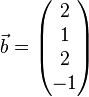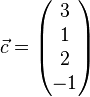und 
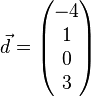linear abhängig sind und überprüfen Sie, welche(r) der Vektoren sich als Linearkombination der jeweils anderen drei Vekotren darstellen lässt/lassen.
Aufgabe 2
a) Prüfen Sie, ob die Vektoren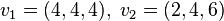 
und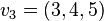 
ein Erzeugendensystem von
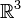bilden.
b) Untersuchen Sie, für welche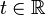 
die Vektoren 
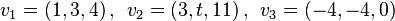linear abhängig in 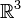sind.
zu b)
ich habe raus, dass für t=50/6 die Vektoren linear unabhängig sind, das heißt für t ungleich 50/6 sind sie linear abhängig. Stimmt das?
Aufgabe 3
Bestimmen Sie eine Basis des von der Menge erzeugenten Vektorraum U=<X>.
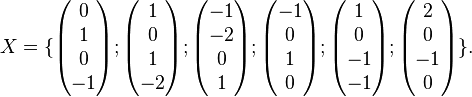
Gilt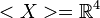 
?
Aufgabe 4
Geben Sie für folgende Vektorräume eine Basis an:
a)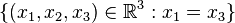 
b)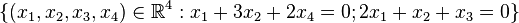
Aufgabe 5
Sei V ein reeler Vektorraum und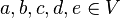 
. Zeigen Sie, dass die folgenden Vektoren linear abgängig sind:
, 
, 
, 
, 
, 
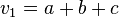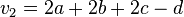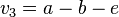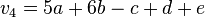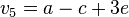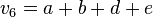
Aufgabe 6
Bestimmen Sie eine Basis des von der Menge erzeugenten Vektorraum U=<X>.
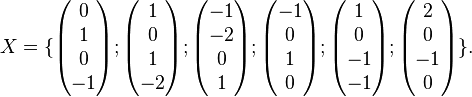
Gilt ?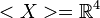
Aufgabe 7
Geben Sie für folgende Vektorräume eine Basis an:
a)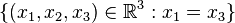
b)
