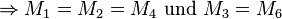Lösung von Aufgabe 1.2 (WS 16 17): Unterschied zwischen den Versionen
Aus Geometrie-Wiki
AlanTu (Diskussion | Beiträge) (Lösung um den Lösungsweg ergänzt.) |
|||
| Zeile 7: | Zeile 7: | ||
<math>M_6 = \{x\vert x\in \mathbb{R}\wedge (x+2)^{2} = 0\}</math><br /><br /> | <math>M_6 = \{x\vert x\in \mathbb{R}\wedge (x+2)^{2} = 0\}</math><br /><br /> | ||
| − | <popup> | + | <popup name="Lösung von AlanTu"> |
| − | <math> | + | <math> |
| + | \begin{align} | ||
| + | && x+2 & = 0 & \|-2\\ | ||
| + | \iff && x & = -2 | ||
| + | \end{align} | ||
| + | </math> | ||
| − | |||
| − | <math> | + | <math>\Rightarrow M_1 = \{x|x\in\mathbb{N}\wedge x = -2\} = \emptyset</math> (da <math>-2\notin\mathbb{N}</math>) |
| − | < | + | <br><hr><br> |
| − | <math> | + | <math> |
| + | \begin{align} | ||
| + | && x^2+2 & = 0 & \|-2 \\ | ||
| + | \iff && x^2 & = -2 & \|\sqrt{\quad} \\ | ||
| + | \iff && x & = \pm i\sqrt{2} | ||
| + | \end{align} | ||
| + | </math> | ||
| − | <math>M_6 = \{-2\}</math> | + | |
| + | <math>\Rightarrow M_2 = \{x\vert x\in \mathbb{R}\wedge x = \pm \sqrt{2}\cdot i\} = \emptyset</math> (da <math>\pm \sqrt{2}\cdot i \notin \mathbb{R}</math>) | ||
| + | |||
| + | <br><hr><br> | ||
| + | |||
| + | <math> | ||
| + | \begin{align} | ||
| + | && x+2 & = 0 & \|-2 \\ | ||
| + | \iff && x & = -2 | ||
| + | \end{align} | ||
| + | </math> | ||
| + | |||
| + | |||
| + | <math>\Rightarrow M_3 = \{x|x\in\mathbb{Z}\wedge x = -2\} = \{-2\}</math> (da <math>-2\in\mathbb{Z}</math>) | ||
| + | |||
| + | <br><hr><br> | ||
| + | |||
| + | <math> | ||
| + | \begin{align} | ||
| + | && x^2-2 & = 0 & \|+2 \\ | ||
| + | \iff && x^2 & = 2 & \|\sqrt{\quad} \\ | ||
| + | \iff && x & = \pm \sqrt{2} | ||
| + | \end{align} | ||
| + | </math> | ||
| + | |||
| + | |||
| + | <math>\Rightarrow M_4 = \{x\vert x\in \mathbb{Q}\wedge x = \pm\sqrt{2}\} = \emptyset</math> (da <math>\sqrt{2}\notin\mathbb{Q}</math>) | ||
| + | |||
| + | <br><hr><br> | ||
| + | |||
| + | <math> | ||
| + | \begin{align} | ||
| + | && x^2-2 & = 0 & \|+2 \\ | ||
| + | \iff && x^2 & = 2 & \|\sqrt{\quad} \\ | ||
| + | \iff && x & = \pm \sqrt{2} | ||
| + | \end{align} | ||
| + | </math> | ||
| + | |||
| + | |||
| + | <math>\Rightarrow M_5 = \{x\vert x\in \mathbb{R}\wedge x = \pm\sqrt{2}\} = \{-\sqrt{2}, \sqrt{2}\}</math> (da <math>\pm\sqrt{2}\in\mathbb{R}</math>) | ||
| + | |||
| + | <br><hr><br> | ||
| + | |||
| + | <math> | ||
| + | \begin{align} | ||
| + | && (x+2)^2 & = 0 & \|\sqrt{\quad} \\ | ||
| + | \iff && x+2 & = 0 & \|-2 \\ | ||
| + | \iff && x & = -2 | ||
| + | \end{align} | ||
| + | </math> | ||
| + | |||
| + | |||
| + | <math>\Rightarrow M_6 = \{x\vert x\in \mathbb{R}\wedge x = -2\} = \{-2\}</math> (da <math>-2\in\mathbb{R}</math>) | ||
| + | |||
| + | <br><hr><br> | ||
<math>\Rightarrow M_1 = M_2 = M_4 \text{ und } M_3 = M_6</math> | <math>\Rightarrow M_1 = M_2 = M_4 \text{ und } M_3 = M_6</math> | ||
</popup>--[[Benutzer:AlanTu|AlanTu]] ([[Benutzer Diskussion:AlanTu|Diskussion]]) 23:53, 20. Okt. 2016 (CEST) | </popup>--[[Benutzer:AlanTu|AlanTu]] ([[Benutzer Diskussion:AlanTu|Diskussion]]) 23:53, 20. Okt. 2016 (CEST) | ||
| − | <popup> | + | <popup name="Lösung von AJWeber"> |
[[Datei:Loesung 1 2 ajweber.JPG|500px]] | [[Datei:Loesung 1 2 ajweber.JPG|500px]] | ||
</popup>--[[Benutzer:AndyWeber|AJWeber]] ([[Benutzer Diskussion:AndyWeber|Diskussion]]) 17:59, 21. Okt. 2016 (CEST) | </popup>--[[Benutzer:AndyWeber|AJWeber]] ([[Benutzer Diskussion:AndyWeber|Diskussion]]) 17:59, 21. Okt. 2016 (CEST) | ||
Version vom 24. Oktober 2016, 21:22 Uhr
Geben Sie eine andere Schreibweise der folgenden Mengen an und prüfen Sie, welche Mengen identisch sind.
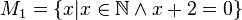
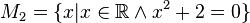
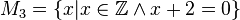
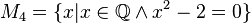
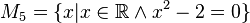
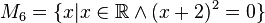

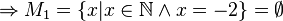 (da
(da 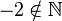 )
)
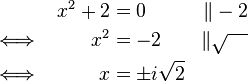
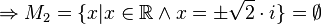 (da
(da 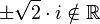 )
)
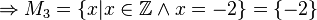 (da
(da  )
)

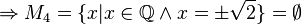 (da
(da 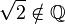 )
)
 (da
(da 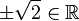 )
)
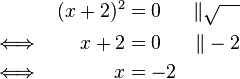
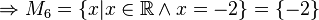 (da
(da 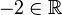 )
)