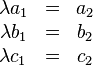Übungsaufgaben zur Algebra, Serie 4 SoSe 2018: Unterschied zwischen den Versionen
Aus Geometrie-Wiki
*m.g.* (Diskussion | Beiträge) (→Aufgabe 4.2) |
*m.g.* (Diskussion | Beiträge) (→Aufgabe 4.2) |
||
| Zeile 9: | Zeile 9: | ||
=Aufgabe 4.2= | =Aufgabe 4.2= | ||
| − | Die Gleichung <math>a_2x+b_2y=c_2</math> ist eine Linearkombination der Gleichung <math>a_1x+b_1y=c_1</math>, wenn eine Zahl <math>\lambda \in \mathbb{R}</math> derart | + | Die Gleichung <math>a_2x+b_2y=c_2</math> ist eine Linearkombination der Gleichung <math>a_1x+b_1y=c_1</math>, wenn eine Zahl <math>\lambda \in \mathbb{R}</math> derart existiert, |
dass <br /> | dass <br /> | ||
<math>\begin{matrix} \lambda a_1 &=& a_2 \\ \lambda b_1 &=& b_2 \\ \lambda c_1 &=& c_2 \end{matrix}</math> <br /> | <math>\begin{matrix} \lambda a_1 &=& a_2 \\ \lambda b_1 &=& b_2 \\ \lambda c_1 &=& c_2 \end{matrix}</math> <br /> | ||
Version vom 12. Mai 2018, 14:26 Uhr
Aufgabe 4.1Wir betrachten auf der Menge der natürlichen Zahlen, die Relationen Teiler und echter Teiler. Aufgabe 4.2Die Gleichung Aufgabe 4.3Aufgabe 4.4Aufgabe 4.5Aufgabe 4.6Aufgabe 4.7Aufgabe 4.8Aufgabe 4.9Aufgabe 4.10 |
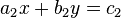 ist eine Linearkombination der Gleichung
ist eine Linearkombination der Gleichung  , wenn eine Zahl
, wenn eine Zahl 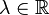 derart existiert,
dass
derart existiert,
dass