Lösung von Aufg. 11.3: Unterschied zwischen den Versionen

Aus Geometrie-Wiki
Wechseln zu: Navigation, Suche
(Die Seite wurde neu angelegt: Beweisen Sie Satz VII.6 a: ::Wenn ein Punkt <math>\ P</math> zu den Endpunkten der Strecke <math>\overline{AB}</math> jeweils ein und denselben Abstand hat, so ist er ...)
 
 
(3 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt)
Zeile 4: Zeile 4:
  
 
[[Category:Einführung_Geometrie]]
 
[[Category:Einführung_Geometrie]]
 +
 +
<p>--------------------------------------------------------------------</p>
 +
 +
<p>'''Voraussetzung:'''Es sei eine Strecke <math> \overline{AB} </math> und ein Punkt P mit <math> \overline{PA} \cong \overline{PB} </math></p><br />
 +
 +
<p>'''Behauptung:''' <math>P \in m</math> , m ist Mittelsenkrechte von <math>\overline{AB}</math></p><br />
 +
 +
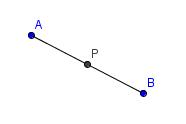
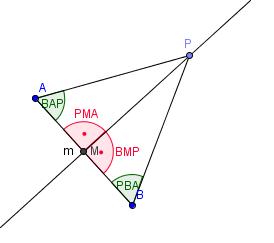
<p>Fall 1: koll(A,B,P)[[Bild:PMPAB.JPG]]<br />Fall 2: nkoll(A,B,P)[[Bild:PAcongPB113.JPG]]</p><br />
 +
 +
 +
 +
 +
{| class="wikitable "
 +
|+ Beweis zu Fall 1
 +
! style="background: #A2CD5A;" |Nr.
 +
! style="background: #A2CD5A;" |Beweisschritt
 +
! style="background: #A2CD5A;" |Begründung
 +
|-
 +
! style="background: #EEE685;"|(I)
 +
| P ist Mittelpunkt von <math>\overline{AB}</math>
 +
| Vor.(<math> \overline{PA} \cong \overline{PB} </math>),Def.III.1 (Mittelpunkt)
 +
|-
 +
! style="background: #EEE685;"|(II)
 +
| <math>P \in m</math>
 +
| I, Def VI.1(Mittelsenkrechte)
 +
|-
 +
 +
 +
|}
 +
 +
{| class="wikitable "
 +
|+ Beweis zu Fall 2
 +
! style="background: #A2CD5A;" |Nr.
 +
! style="background: #A2CD5A;" |Beweisschritt
 +
! style="background: #A2CD5A;" |Begründung
 +
|-
 +
! style="background: #EEE685;"|(I)
 +
| <math>\triangle ABP</math> ist gleichschenklig
 +
| Vor.(<math> \overline{PA} \cong \overline{PB} </math>), Def.VII.4 (gleichschenkliges Dreieck)
 +
|-
 +
! style="background: #EEE685;"|(II)
 +
| <math>\angle PBA \cong \angle BAP</math>
 +
| I, Satz VII.5 (Basiswinkelsatz)
 +
|-
 +
! style="background: #EEE685;"|(III)
 +
| <math>\exists ! M \in \overline{AB} : \overline{MA} \cong \overline{MB}</math>
 +
| Satz III.1(Existenz und Eindeutigkeit des Mittelpunkts), Def.III.1 (Mittelpunkt)
 +
|-
 +
! style="background: #EEE685;"|(IV)
 +
| <math>\triangle AMP \cong \triangle MBP</math>
 +
| II, III, Vor.(<math> \overline{PA} \cong \overline{PB} </math>), Axiom V (SWS)
 +
|-
 +
! style="background: #EEE685;"|(V)
 +
| <math>\angle PMA \cong \angle BMP</math> 
 +
| IV, Def.VII.3 (Dreieckskongruenz)
 +
|-
 +
! style="background: #EEE685;"|(VI)
 +
| <math>\angle PMA , \angle BMP</math> sind Nebenwinkel
 +
| IV, Def.V.4 (Nebenwinkel)
 +
|-
 +
! style="background: #EEE685;"|(VII)
 +
| <math>| \angle PMA | = | \angle BMP | = 90^{\circ}</math> 
 +
| V, VI, Def V.6 (rechter Winkel)
 +
|-
 +
! style="background: #EEE685;"|(VIII)
 +
| <math>\overline{MP} \bot \overline{AB}</math> 
 +
| VII, Def.V.9 (noch mehr Senkrecht)
 +
|-
 +
! style="background: #EEE685;"|(IX)
 +
| <math>\overline{MP} \subset m</math> 
 +
| III, VIII, Def.VI.1 (Mittelsenkrechte)
 +
|-
 +
! style="background: #EEE685;"|(X)
 +
| <math>P \in m</math> 
 +
| IX
 +
|-
 +
 +
|}
 +
 +
'''qed.'''<br />
 +
 +
--[[Benutzer:Studentxyz|Studentxyz]] 17:58, 17. Jan. 2011 (UTC)
 +
<p>--------------------------------------------------------------------</p>
 +
klasse Beweis, sehr schön!--[[Benutzer:Schnirch|Schnirch]] 14:06, 25. Jan. 2011 (UTC)

Aktuelle Version vom 25. Januar 2011, 16:06 Uhr

Beweisen Sie Satz VII.6 a:

Wenn ein Punkt 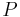\ P zu den Endpunkten der Strecke \overline{AB} jeweils ein und denselben Abstand hat, so ist er ein Punkt der Mittelsenkrechten von \overline{AB}.

--------------------------------------------------------------------

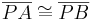
Voraussetzung:Es sei eine Strecke \overline{AB} und ein Punkt P mit \overline{PA} \cong \overline{PB}


Behauptung: 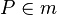P \in m , m ist Mittelsenkrechte von \overline{AB}


Fall 1: koll(A,B,P)PMPAB.JPG
Fall 2: nkoll(A,B,P)PAcongPB113.JPG




Beweis zu Fall 1
Nr. Beweisschritt Begründung
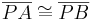(I) P ist Mittelpunkt von \overline{AB} Vor.( \overline{PA} \cong \overline{PB} ),Def.III.1 (Mittelpunkt)
(II) 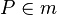P \in m I, Def VI.1(Mittelsenkrechte)
Beweis zu Fall 2
Nr. Beweisschritt Begründung
(I) 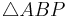\triangle ABP ist gleichschenklig Vor.(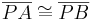 \overline{PA} \cong \overline{PB} ), Def.VII.4 (gleichschenkliges Dreieck)
(II) 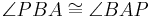\angle PBA \cong \angle BAP I, Satz VII.5 (Basiswinkelsatz)
(III) 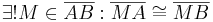\exists ! M \in \overline{AB} : \overline{MA} \cong \overline{MB} Satz III.1(Existenz und Eindeutigkeit des Mittelpunkts), Def.III.1 (Mittelpunkt)
(IV) 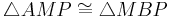\triangle AMP \cong \triangle MBP II, III, Vor.(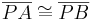 \overline{PA} \cong \overline{PB} ), Axiom V (SWS)
(V) 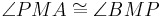\angle PMA \cong \angle BMP IV, Def.VII.3 (Dreieckskongruenz)
(VI) 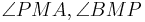\angle PMA , \angle BMP sind Nebenwinkel IV, Def.V.4 (Nebenwinkel)
(VII) | 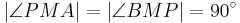\angle PMA | = | \angle BMP | = 90^{\circ} V, VI, Def V.6 (rechter Winkel)
(VIII) 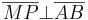\overline{MP} \bot \overline{AB} VII, Def.V.9 (noch mehr Senkrecht)
(IX) 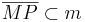\overline{MP} \subset m III, VIII, Def.VI.1 (Mittelsenkrechte)
(X) 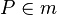P \in m IX

qed.

--Studentxyz 17:58, 17. Jan. 2011 (UTC)

--------------------------------------------------------------------

klasse Beweis, sehr schön!--Schnirch 14:06, 25. Jan. 2011 (UTC)
Von „http://geometrie.zum.de/index.php?title=Lösung_von_Aufg._11.3&oldid=6155“