Serie 05 12 13: Unterschied zwischen den Versionen
Aus Geometrie-Wiki
*m.g.* (Diskussion | Beiträge) (→Aufgabe 5.2) |
K (→Aufgabe 5.3) |
||
| (11 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt) | |||
| Zeile 10: | Zeile 10: | ||
=Aufgabe 5.2= | =Aufgabe 5.2= | ||
| − | Es sei <math>Z=\begin{pmatrix} 0 \\ 0 \\ d \end{pmatrix}</math> | + | Es sei <math>Z=\begin{pmatrix} 0 \\ 0 \\ d \end{pmatrix}</math>,<math>d \in \mathbb{R}, d > 0</math> |
| + | |||
| + | Es sei <math>\varepsilon</math> die <math>x-y-</math>Ebene, die wir wiederum als <math>\mathbb{R}^2</math> interpretieren. Wir bilden jedes Element des <math>\mathbb{R}^3</math> mittels der Abbildung <math>ZP_Z</math>auf <math>\varepsilon</math> wie folgt ab:<br /> | ||
| + | <math>\forall P=\begin{pmatrix} x \\ y \\ z \end{pmatrix} \in \mathbb{R} \setminus Z: ZP_Z(P)=ZP\cap \varepsilon</math>.<br /> | ||
| + | Beweisen Sie: <math>ZP_Z</math> ist linear. | ||
| + | |||
| + | =Aufgabe 5.3= | ||
| + | Geben sei <math>B</math> eine Menge, die aus folgenden Vektoren des <math>\mathbb{R}3</math> besteht:<br /> | ||
| + | |||
| + | <math>\vec{b_1}=\begin{pmatrix}2 \\ 0 \\ 0\end{pmatrix}</math>, <math>\vec{b_2}=\begin{pmatrix}2 \\ 2 \\ 0\end{pmatrix}</math>, <math>\vec{b_3}=\begin{pmatrix}0 \\ 0 \\ 3\end{pmatrix}</math> | ||
| + | |||
| + | Beweisen Sie: Jedes <math>\vec{a}</math> aus <math>\mathbb{R}^3</math> lässt sich als Linearkombination der Vektoren der Menge <math>M</math> darstellen. | ||
Aktuelle Version vom 6. Januar 2013, 18:04 Uhr
Aufgabe 5.1
Es sei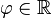 
.
Wir definieren die folgende Abbildung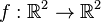 
.
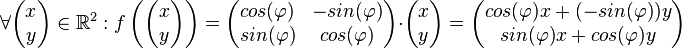Beweisen Sie: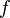 
ist eine lineare Abbildung.
Interpretieren Sie 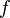geometrisch.
Hilfe:
Aufgabe 5.2
Es sei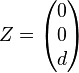 
,
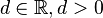
Es sei 
die 
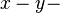Ebene, die wir wiederum als 
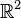interpretieren. Wir bilden jedes Element des 
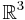mittels der Abbildung 
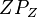auf wie folgt ab:
.
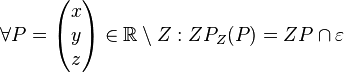Beweisen Sie: 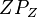ist linear.
Aufgabe 5.3
Geben sei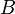 
eine Menge, die aus folgenden Vektoren des 
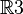besteht:
, 
, 
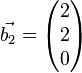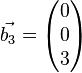
Beweisen Sie: Jede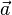s 
aus 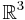lässt sich als Linearkombination der Vektoren der Menge 
darstellen.
