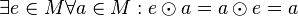Gruppendefinition (lang): Unterschied zwischen den Versionen
Aus Geometrie-Wiki
*m.g.* (Diskussion | Beiträge) (→Definition 2: (Halbgruppe)) |
*m.g.* (Diskussion | Beiträge) (→Definition 2: (Halbgruppe)) |
||
| Zeile 14: | Zeile 14: | ||
#(Abgeschlossenheit) <math>\forall a,b \in H: a \odot b \in H</math> | #(Abgeschlossenheit) <math>\forall a,b \in H: a \odot b \in H</math> | ||
#(Assoziativität) <math>\forall a, b, c: (a \odot b) \odot a = a \odot (b \odot c)</math>. | #(Assoziativität) <math>\forall a, b, c: (a \odot b) \odot a = a \odot (b \odot c)</math>. | ||
| + | =Definition 3: (Monoid)= | ||
| + | Eine Halbgruppe <math>[M, \odot]</math> heißt Monoid, wenn sie ein Einselement hat:<br /> | ||
| + | *(Einselement) <math>\exists e \in M \forall a \in M: e \odot a = a \odot e = a</math> | ||
<!--- Was hier drunter steht muss stehen bleiben ---> | <!--- Was hier drunter steht muss stehen bleiben ---> | ||
|} | |} | ||
</div> | </div> | ||
[[Kategorie:Algebra]] | [[Kategorie:Algebra]] | ||
Version vom 5. November 2017, 17:48 Uhr
Definition 1: (Algebraische Struktur)Eine Menge Schreibweise: Definition 2: (Halbgruppe)Eine algebraische Struktur
Definition 3: (Monoid)Eine Halbgruppe
|
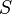 zusammen mit einer Operation
zusammen mit einer Operation  oder Relation
oder Relation  auf dieser Menge nennt man algebraische Struktur.
auf dieser Menge nennt man algebraische Struktur. ![[S, o]](/images/math/8/2/2/8222873ff784df1f8e8e2e6ac14800d4.png) bzw
bzw ![[S, r]](/images/math/8/9/e/89ec8cf7ec3eaea8e94ef96163bb1340.png)
![[H, \odot]](/images/math/b/5/0/b5051dd155dce8001fa2c6d3eec0868f.png) heißt Halbgruppe, wenn
heißt Halbgruppe, wenn
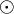 auf
auf 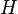 abgeschlossen und assoziativ ist.
abgeschlossen und assoziativ ist.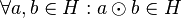
 .
.
![[M, \odot]](/images/math/1/b/7/1b76d279ea0a66347bff1d26e034943a.png) heißt Monoid, wenn sie ein Einselement hat:
heißt Monoid, wenn sie ein Einselement hat: