Übungen 03: Unterschied zwischen den Versionen
Aus Geometrie-Wiki
(→Aufgabe 2) |
(→Aufgabe 1) |
||
| Zeile 10: | Zeile 10: | ||
hat.<br /> | hat.<br /> | ||
Was bedeutet das anschaulich für die Lage der Ebenen? | Was bedeutet das anschaulich für die Lage der Ebenen? | ||
| + | |||
| + | =Aufgabe 2= | ||
| + | |||
| + | Berechnen Sie mit Hilfe des Gauß-Algorithmus den Schnittpunkt zwischen Gerade und Ebene:<br /> | ||
| + | a) <math>g: \begin{pmatrix} x \\ y \\ z \end{pmatrix} = \begin{pmatrix} 2 \\ 2 \\ 1 \end{pmatrix}+r\begin{pmatrix} 1 \\ -1 \\ 1 \end{pmatrix} </math> und <math>e: \begin{pmatrix} x \\ y \\ z \end{pmatrix} = \begin{pmatrix} 1\\ 1 \\ 5 \end{pmatrix}+s\begin{pmatrix} 2 \\ 0 \\ 1 \end{pmatrix}+t\begin{pmatrix} -1 \\ -1 \\ 3 \end{pmatrix}</math> | ||
| + | |||
| + | b) folgt. | ||
=Aufgabe 2= | =Aufgabe 2= | ||
Version vom 29. April 2013, 17:25 Uhr
Inhaltsverzeichnis |
Aufgabe 1
Gegeben sind drei Ebenengleichungen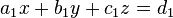 
, 
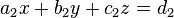und 
.
Geben Sie drei Ebenen dieser Form an, sodass das LGS dazu
a) genau eine Lösung
b) keine Lösung
c) eine ein-parametrige Lösung
d) eine zwei-parametrige Lösung
hat.
Was bedeutet das anschaulich für die Lage der Ebenen?
Aufgabe 2
Berechnen Sie mit Hilfe des Gauß-Algorithmus den Schnittpunkt zwischen Gerade und Ebene:
a)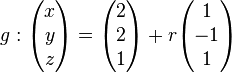 
und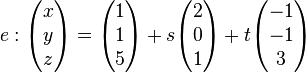 
b) folgt.
Aufgabe 2
Bestimmen Sie ein lineares Gleichungssystem (bestehend aus zwei Gleichungen), das die Gerade durch die Punkte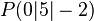 
und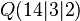 
beschreibt. Tipp: Überlegen Sie sich, was das geometrisch repräsentiert.
