Natürliche Zahlen mit Multiplikation: Unterschied zwischen den Versionen

Aktuelle Version vom 9. Juli 2018, 18:37 Uhr

Wir überprüfen ob es sich bei $[\N,\sdot]$ um eine Gruppe handelt:

(1) Abgeschlossenheit

Beispiel: 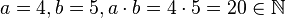$a=4, b=5, a \sdot b=4 \sdot 5=20 \in \N$ passt.

(2) Assoziativität

Beispiel: 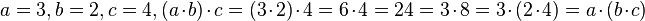$a=3, b=2, c=4, (a \sdot b)\sdot c=(3 \sdot 2) \sdot 4=6 \sdot 4=24=3 \sdot 8=3 \sdot (2 \sdot 4)=a \sdot (b \sdot c)$ passt.

(3) neutrales Element

Beispiel: 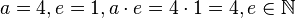$a=4, e=1, a \sdot e=4 \sdot 1=4, e \in \N$ passt.

(4) inverses Element

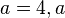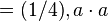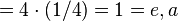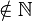Beispiel: $a=4, a$ ^-1 $=(1/4), a \sdot a$ ^-1 $=4 \sdot (1/4)=1=e, a$ ^-1 $\notin \N$ passt nicht!

Somit handelt es sich bei $[\N,\sdot]$ nicht um eine Gruppe.

@@ Zeile 16: / Zeile 16: @@
 (4) inverses Element
-Beispiel: <math> a=4, a</math><sup>-1</sup><math>=(1/4), a \sdot a</math><sup>-1</sup><math>=4 \sdot (1/4)=0, a</math><sup>-1</sup><math> \notin \N </math> <span style="color:red;">passt nicht!</span>
+Beispiel: <math> a=4, a</math><sup>-1</sup><math>=(1/4), a \sdot a</math><sup>-1</sup><math>=4 \sdot (1/4)=1=e, a</math><sup>-1</sup><math> \notin \N </math> <span style="color:red;">passt nicht!</span>
 Somit handelt es sich bei <math>[\N,\sdot]</math> nicht um eine Gruppe.