Lösung von Aufg. 6.3P (WS 18 19): Unterschied zwischen den Versionen
Aus Geometrie-Wiki
(Die Seite wurde neu angelegt: „Beweisen Sie: Der Durchschnitt zweier konvexer Punktmengen ist konvex. Kategorie:Geo_P“) |
CIG UA (Diskussion | Beiträge) |
||
| Zeile 2: | Zeile 2: | ||
| + | 1. M <span>∩</span> N = O, mit M, N, O sind konvex<br /> | ||
| + | 2. {A,B | <math>\overline{AB}</math> ε M} <span>∩</span> {C,D | <math>\overline{CD}</math> ε M} <=> O<br /> | ||
| + | 3. O = {A,B,C,D | <math>\overline{AB}</math> ε M <math>\wedge</math> <math>\overline{AB}</math> ε N <math>\wedge</math> <math>\overline{CD}</math> ε M <math>\wedge</math> <math>\overline{CD}</math> ε N}<br /> | ||
| + | 4. O = {A,B,C,D | <math>\overline{AB}</math><math>\wedge</math> <math>\overline{AC}</math><math>\wedge</math> <math>\overline{AD}</math><math>\wedge</math> <math>\overline{BC}</math><math>\wedge</math> <math>\overline{BD}</math><math>\wedge</math> <math>\overline{CD}</math> ε O}<br /> | ||
| + | 5. O ist konvex. | ||
| + | |||
| + | Begründung von Schritt 4 -> Transitivität der Korrelation "kongruente (Schnitt)menge" --[[Benutzer:CIG UA|CIG UA]] ([[Benutzer Diskussion:CIG UA|Diskussion]]) 12:52, 23. Nov. 2018 (CET) | ||
[[Kategorie:Geo_P]] | [[Kategorie:Geo_P]] | ||
Version vom 23. November 2018, 12:52 Uhr
Beweisen Sie: Der Durchschnitt zweier konvexer Punktmengen ist konvex.
1. M ∩ N = O, mit M, N, O sind konvex
2. {A,B |  ε M} ∩ {C,D |
ε M} ∩ {C,D | 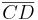 ε M} <=> O
ε M} <=> O
3. O = {A,B,C,D |  ε M
ε M 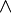
 ε N
ε N 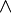
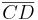 ε M
ε M 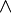
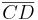 ε N}
ε N}
4. O = {A,B,C,D | 
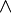
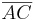
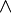

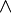
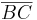
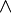

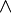
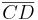 ε O}
ε O}
5. O ist konvex.
Begründung von Schritt 4 -> Transitivität der Korrelation "kongruente (Schnitt)menge" --CIG UA (Diskussion) 12:52, 23. Nov. 2018 (CET)

