Lösung von Aufgabe 13.1P (WS 18/19): Unterschied zwischen den Versionen
Aus Geometrie-Wiki
(Die Seite wurde neu angelegt: „Zeigen Sie, dass bei der Verkettung einer Schubspiegelung <math>G_{a,b,c}</math> (<math>a \parallel b \wedge a \perp c</math>) mit einer Spiegelung <math>S_d</…“) |
CIG UA (Diskussion | Beiträge) |
||
| Zeile 1: | Zeile 1: | ||
Zeigen Sie, dass bei der Verkettung einer Schubspiegelung <math>G_{a,b,c}</math> (<math>a \parallel b \wedge a \perp c</math>) mit einer Spiegelung <math>S_d</math> (<math>d \perp c</math>) eine Punktspiegelung entsteht. | Zeigen Sie, dass bei der Verkettung einer Schubspiegelung <math>G_{a,b,c}</math> (<math>a \parallel b \wedge a \perp c</math>) mit einer Spiegelung <math>S_d</math> (<math>d \perp c</math>) eine Punktspiegelung entsteht. | ||
<br /> | <br /> | ||
| − | + | <br /> | |
| + | <br /> | ||
| + | Vor: S<sub>a</sub><math>\circ</math>S<sub>b</sub><math>\circ</math>S<sub>c</sub><math>\circ</math>S<sub>d</sub> mit a || b und a senkrecht zu c und d senkrecht zu c; Beh: = D<sub>P,180</sub><br /> | ||
| + | 1.) = S<sub>c</sub><math>\circ</math>S<sub>a</sub><math>\circ</math>S<sub>b</sub><math>\circ</math>S<sub>d</sub> '''- b || d (Vor.); Eigenschaft Schubspiegelung'''<br /> | ||
| + | 2.) = S<sub>c</sub><math>\circ</math>S<sub>a</sub><math>\circ</math>S<sub>b'</sub><math>\circ</math>S<sub>d'</sub> mit a=b' und b' || d' und |bd| = |b'd'| '''- 1.) Eig. Verschiebung'''<br /> | ||
| + | 3.) = S<sub>c</sub><math>\circ</math>S<sub>d'</sub><math> '''- 2.), Identität a,b''''<br /> | ||
| + | 4.) = D<sub>P,180</sub> mit c geschnitten d' = P<br /> | ||
| + | Die Behauptung ist bewiesen. --~~~~ | ||
[[Kategorie:Geo_P]] | [[Kategorie:Geo_P]] | ||
Version vom 25. Januar 2019, 13:08 Uhr
Zeigen Sie, dass bei der Verkettung einer Schubspiegelung 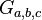 (
(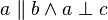 ) mit einer Spiegelung
) mit einer Spiegelung 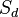 (
(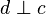 ) eine Punktspiegelung entsteht.
) eine Punktspiegelung entsteht.
Vor: Sa Sb
Sb Sc
Sc Sd mit a || b und a senkrecht zu c und d senkrecht zu c; Beh: = DP,180
Sd mit a || b und a senkrecht zu c und d senkrecht zu c; Beh: = DP,180
1.) = Sc Sa
Sa Sb
Sb Sd - b || d (Vor.); Eigenschaft Schubspiegelung
Sd - b || d (Vor.); Eigenschaft Schubspiegelung
2.) = Sc Sa
Sa Sb'
Sb' Sd' mit a=b' und b' || d' und |bd| = |b'd'| - 1.) Eig. Verschiebung
Sd' mit a=b' und b' || d' und |bd| = |b'd'| - 1.) Eig. Verschiebung
3.) = Sc Sd'Fehler beim Parsen(Lexikalischer Fehler): '''- 2.), Identität a,b''''<br /> 4.) = D<sub>P,180</sub> mit c geschnitten d' = P<br /> Die Behauptung ist bewiesen. --~~~~ [[Kategorie:Geo_P]]
Sd'Fehler beim Parsen(Lexikalischer Fehler): '''- 2.), Identität a,b''''<br /> 4.) = D<sub>P,180</sub> mit c geschnitten d' = P<br /> Die Behauptung ist bewiesen. --~~~~ [[Kategorie:Geo_P]]

