Lösung von Aufg. 7.4: Unterschied zwischen den Versionen
| Zeile 19: | Zeile 19: | ||
5)<math>A \notin\delta_2 </math>________________wegen nkomp(A,B,C,D)<br /> | 5)<math>A \notin\delta_2 </math>________________wegen nkomp(A,B,C,D)<br /> | ||
6)<math>B \in\delta_2 </math> und <math>B \in\epsilon </math>____________2) und 4)<br /> | 6)<math>B \in\delta_2 </math> und <math>B \in\epsilon </math>____________2) und 4)<br /> | ||
| − | 7)<math>\exists P</math> ,<math>P \in\epsilon </math>,<math> | + | 7)<math>\exists P</math> ,<math>P \in\epsilon </math>,<math>P \in\delta_2 </math>________6) und Axiom I/6 |
bleibt zu zeigen : <math>A\not\equiv P</math><br /> | bleibt zu zeigen : <math>A\not\equiv P</math><br /> | ||
Version vom 17. Dezember 2010, 16:23 Uhr
Beweisen Sie: Jede Ebene enthält wenigstens drei paarweise verschiedene Punkte.
Vor: Ebene  ,nkomp(A,B,C,D)
,nkomp(A,B,C,D)
Beh:  enthält weinigstens drei paarweise verschiedene Punkte
enthält weinigstens drei paarweise verschiedene Punkte
Fall 1:
3 der vier Punkte liegen in der Ebene 
 trivial
trivial
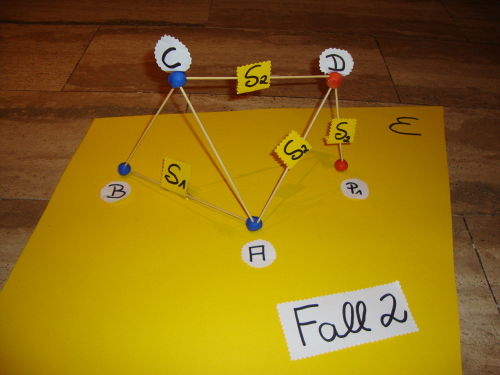
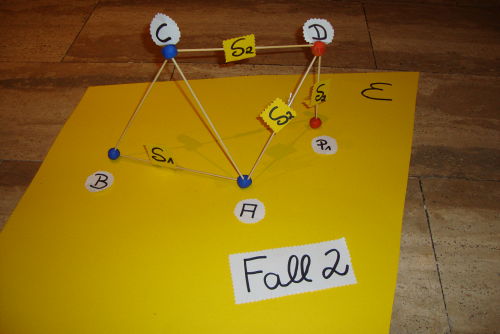
Fall 2:
2 der vier Punkte liegen in der Ebene 
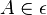 ,
,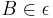
1) 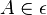 ,
, 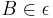 ,
, 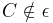 und
und 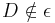
2)
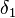 ________Lemma 3 und Axiom I/4
________Lemma 3 und Axiom I/4
3)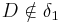 __________________wegen nkomp(A,B,C,D)
__________________wegen nkomp(A,B,C,D)
4)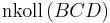
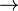
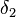 ___________3)
___________3)
5)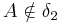 ________________wegen nkomp(A,B,C,D)
________________wegen nkomp(A,B,C,D)
6)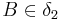 und
und 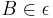 ____________2) und 4)
____________2) und 4)
7)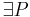 ,
, ,
,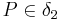 ________6) und Axiom I/6
________6) und Axiom I/6
bleibt zu zeigen : 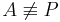
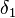 :
: 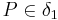 ,
, 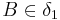 ,
, 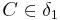
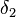 :
: 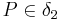 ,
, 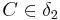 ,
, 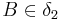
daraus folgt 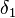

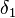
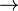 komp(A,B,C,D)
komp(A,B,C,D)
8) Widerspruch zur Vorraussetzung nkomp(A,B,C,D)
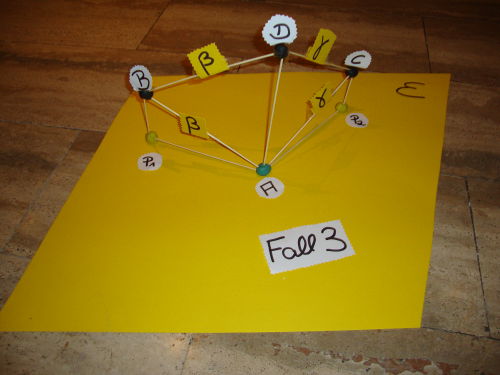
3.Fall:
1)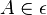
2)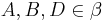 __________________Axiom I/4 und Lemma 3
__________________Axiom I/4 und Lemma 3
3)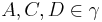 ________________Axiom I/4 und Lemma 3
________________Axiom I/4 und Lemma 3
4)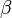
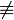
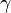 _____________da sonst
_____________da sonst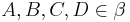 Widerspruch zur nkomp(A,B,C,D)
Widerspruch zur nkomp(A,B,C,D)
5)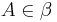 ,
, 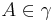 __________2) und 3)
__________2) und 3)
6)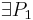 ,
, 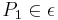 ,
, 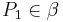 ___________Axiom I/4
___________Axiom I/4
7)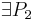 ,
, 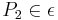 ,
, 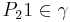 ___________Axiom I/4
___________Axiom I/4
zu zeigen: 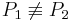
Annahme: P1 =P2
8) A, D und 
9) A, D und 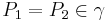
10)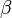 =
= 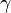
11) Widerspruch zu 4)
 A,P1,P2 sind drei paarweise verschiedene Punkte in
A,P1,P2 sind drei paarweise verschiedene Punkte in 
Fall 4:
Keine der vier Punkte ist Element von 
 enthält einen Punkt________nach Axiom I/4
enthält einen Punkt________nach Axiom I/4
 Fall 3
Fall 3