Lösung von Aufg. 13.3: Unterschied zwischen den Versionen
| Zeile 1: | Zeile 1: | ||
Man beweise: Ein Punkt <math>\ P</math> gehört genau dann zur Winkelhalbierenden des Winkels <math>\ \alpha</math>, wenn er zu den Schenkeln von <math>\ \alpha</math> jeweils denselben Abstand hat. | Man beweise: Ein Punkt <math>\ P</math> gehört genau dann zur Winkelhalbierenden des Winkels <math>\ \alpha</math>, wenn er zu den Schenkeln von <math>\ \alpha</math> jeweils denselben Abstand hat. | ||
| − | Vor: <math>P \in w</math>, , <math>\angle ASP</math> <math>\cong</math><math>\angle PSB</math><br /> | + | <u>Vor</u>: <math>P \in w</math>, , <math>\angle ASP</math> <math>\cong</math><math>\angle PSB</math><br /> |
| − | Beh: <math>\overline {AP}</math> <math>\cong</math><math>\overline {BP}</math><br /> | + | <u>Beh:</u> <math>\overline {AP}</math> <math>\cong</math><math>\overline {BP}</math><br /> |
1)<math>\angle ASP</math> <math>\cong</math><math>\angle PSB</math> __________________Vor<br /> | 1)<math>\angle ASP</math> <math>\cong</math><math>\angle PSB</math> __________________Vor<br /> | ||
| − | 2)Lote werden durch P auf die jeweiligen Schenkel des Winkels________________Existenz und Eindeutigkeit des Lotes<br /> | + | 2) Lote werden durch P auf die jeweiligen Schenkel des Winkels________________Existenz und Eindeutigkeit des Lotes<br /> |
<math>\angle ASB</math> gefällt<br /> | <math>\angle ASB</math> gefällt<br /> | ||
3)|<math>\angle {SAP}</math>| =|<math>\angle {SBP}</math>| =90________________2)<br /> | 3)|<math>\angle {SAP}</math>| =|<math>\angle {SBP}</math>| =90________________2)<br /> | ||
| Zeile 14: | Zeile 14: | ||
| − | Vor:<math>\overline {AP}</math> <math>\cong</math><math>\overline {BP}</math><br /> | + | <u>Vor</u>:<math>\overline {AP}</math> <math>\cong</math><math>\overline {BP}</math><br /> |
| − | Beh: <math>P \in w</math>, <math>\angle ASP</math> <math>\cong</math><math>\angle PSB</math><br /> | + | <u>Beh</u>: <math>P \in w</math>, <math>\angle ASP</math> <math>\cong</math><math>\angle PSB</math><br /> |
1)<math>\overline {AP}</math><math>\cong</math> <math>\overline {BP}</math>___________________Vor.<br /> | 1)<math>\overline {AP}</math><math>\cong</math> <math>\overline {BP}</math>___________________Vor.<br /> | ||
| − | 2)Lote werden durch P auf die jeweiligen Schenkel des Winkels________________Existenz und Eindeutigkeit des Lotes<br /> | + | 2) Lote werden durch P auf die jeweiligen Schenkel des Winkels________________Existenz und Eindeutigkeit des Lotes<br /> |
<math>\angle ASB</math> gefällt<br /> | <math>\angle ASB</math> gefällt<br /> | ||
3)|<math>\angle {SAP}</math>| =|<math>\angle {SBP}</math>| =90_________________2)<br /> | 3)|<math>\angle {SAP}</math>| =|<math>\angle {SBP}</math>| =90_________________2)<br /> | ||
Version vom 25. Januar 2011, 19:38 Uhr
Man beweise: Ein Punkt 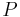 gehört genau dann zur Winkelhalbierenden des Winkels
gehört genau dann zur Winkelhalbierenden des Winkels 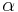 , wenn er zu den Schenkeln von
, wenn er zu den Schenkeln von 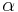 jeweils denselben Abstand hat.
jeweils denselben Abstand hat.
Vor: 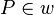 , ,
, , 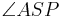
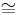
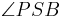
Beh: 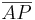
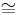
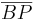
1)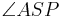
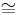
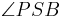 __________________Vor
__________________Vor
2) Lote werden durch P auf die jeweiligen Schenkel des Winkels________________Existenz und Eindeutigkeit des Lotes
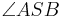 gefällt
gefällt
3)|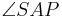 | =|
| =|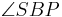 | =90________________2)
| =90________________2)
4)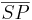 =
= 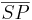 ___________________trivial
___________________trivial
5)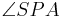
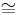
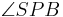 __________________1), 2) und Innenwinkelsumme im Dreieck
__________________1), 2) und Innenwinkelsumme im Dreieck
6)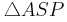
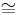
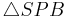 ______________WSW,1), 4),5)
______________WSW,1), 4),5)
7) 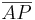 =
= 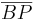 ______________________6)--Engel82 17:22, 25. Jan. 2011 (UTC)
______________________6)--Engel82 17:22, 25. Jan. 2011 (UTC)
Vor: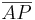
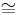
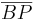
Beh: 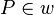 ,
, 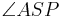
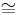
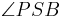
1)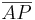
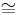
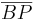 ___________________Vor.
___________________Vor.
2) Lote werden durch P auf die jeweiligen Schenkel des Winkels________________Existenz und Eindeutigkeit des Lotes
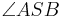 gefällt
gefällt
3)|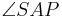 | =|
| =|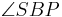 | =90_________________2)
| =90_________________2)
4)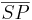
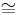
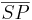 ___________________trivial
___________________trivial
5)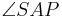
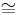
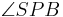 __________________SsW, 1),3),4) und Satz:der größeren Seite liegt der größere Winkel gegenüber.
__________________SsW, 1),3),4) und Satz:der größeren Seite liegt der größere Winkel gegenüber.
6)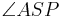
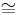
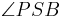 _________________________5)
_________________________5)
7) 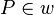 , ____________________6)--Engel82 17:33, 25. Jan. 2011 (UTC)
, ____________________6)--Engel82 17:33, 25. Jan. 2011 (UTC)

