Lösung von Aufg. 12.3 SS11: Unterschied zwischen den Versionen

Aus Geometrie-Wiki
Wechseln zu: Navigation, Suche
Zeile 35: Zeile 35:
 
<math>\ 6) \ y1 \ = \ y2 \ (3)</math><br>
 
<math>\ 6) \ y1 \ = \ y2 \ (3)</math><br>
 
<math>\ 7) \angle \ PAB \cong \angle \ PBA \ (1, \ Basiswinkelsatz)</math><br>
 
<math>\ 7) \angle \ PAB \cong \angle \ PBA \ (1, \ Basiswinkelsatz)</math><br>
−
<math>\ 8) \triangle \ APP2 \cong \triangle \ BPP2 \ (SWS, \ 5, \ 6, \ 7)</math><br>
+
<math>\ 8) \triangle \ APP2 \cong \triangle \ BPP2 \ (WSW, \ 5, \ 6, \ 7)</math><br>
 
<math>\ 9) \angle | \ AP2P  | \ = \angle | \ BP2P | \ = \ 90 </math> (8, Def. Nebenwinkel, Supplementaxiom)<br>
 
<math>\ 9) \angle | \ AP2P  | \ = \angle | \ BP2P | \ = \ 90 </math> (8, Def. Nebenwinkel, Supplementaxiom)<br>
 
<math>\ 10) | \overline{AP2} | \ = | \overline{BP2}</math>  (8) <br>
 
<math>\ 10) | \overline{AP2} | \ = | \overline{BP2}</math>  (8) <br>

Version vom 5. Juli 2011, 14:13 Uhr

Beweisen Sie Satz VII.6 a:

Wenn ein Punkt 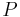\ P zu den Endpunkten der Strecke \overline{AB} jeweils ein und denselben Abstand hat, so ist er ein Punkt der Mittelsenkrechten von \overline{AB}.


\ Beweis:

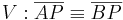
\ V: \overline{AP} \equiv \overline{BP}
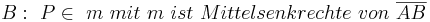
\ B: \ P\in \ m \ mit \ m \ ist \ Mittelsenkrechte \ von \ \overline{AB}

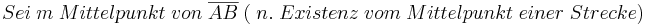
\ Sei \ m \ Mittelpunkt \ von \ \overline{AB}\ ( \ n. \ Existenz \ vom \ Mittelpunkt \ einer \ Strecke)
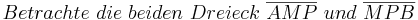
\ Betrachte \ die \ beiden \ Dreieck \ \overline{AMP}\ und\ \overline{MPB}
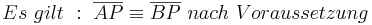
\ Es \ gilt \ : \ \overline{AP} \equiv \overline{BP}\ nach \ Voraussetzung\
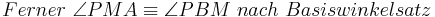
\ Ferner\ \angle PMA \equiv \angle PBM \ nach \ Basiswinkelsatz
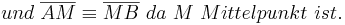
\ und \ \overline{AM} \equiv \overline{MB} \ da \ M \ Mittelpunkt\ ist.
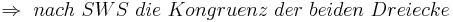
\Rightarrow \ nach \ SWS \ die \ Kongruenz \ der \ beiden \ Dreiecke
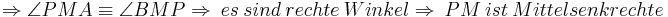
\Rightarrow \angle PMA\equiv \angle BMP\Rightarrow \ es \ sind \ rechte \ Winkel \Rightarrow \ PM \ ist \ Mittelsenkrechte
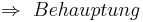\Rightarrow \ Behauptung--Peterpummel 17:47, 3. Jul. 2011 (CEST)

Lösungsvorschlag 2:

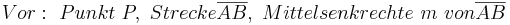
\ Vor: \ Punkt \ P, \ Strecke \overline{AB}, \ Mittelsenkrechte \ m \ von \overline{AB}
|\ PA | = | \ PB |
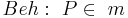
\ Beh: \ P \in \ m

Man muss in zwei Fälle unterscheiden:
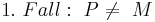
\ 1. \ Fall: \ P \neq \ M
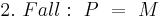
\ 2. \ Fall: \ P \ = \ M

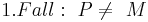
\ 1. Fall: \ P \neq \ M
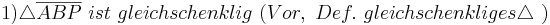
\ 1) \triangle \overline{ABP} \ ist \ gleichschenklig \ (Vor, \ Def. \ gleichschenkliges \triangle \ )
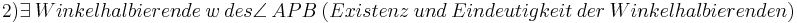
\ 2) \exists \ Winkelhalbierende \ w \ des \angle \ APB \ (Existenz \ und \ Eindeutigkeit \ der \ Winkelhalbierenden)
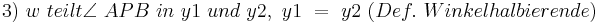
\ 3) \ w \ teilt \angle \ APB \ in \ y1 \ und \ y2, \ y1 \ = \ y2 \ (Def. \ Winkelhalbierende)
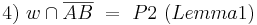
\ 4) \ w \cap \overline{AB} \ = \ P2 \ (Lemma1)
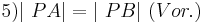
\ 5)|\ PA | = | \ PB | \ (Vor.)
\ 6) \ y1 \ = \ y2 \ (3)
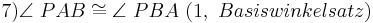
\ 7) \angle \ PAB \cong \angle \ PBA \ (1, \ Basiswinkelsatz)
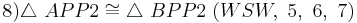
\ 8) \triangle \ APP2 \cong \triangle \ BPP2 \ (WSW, \ 5, \ 6, \ 7)
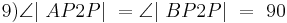\ 9) \angle | \ AP2P | \ = \angle | \ BP2P | \ = \ 90 (8, Def. Nebenwinkel, Supplementaxiom)
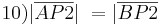\ 10) | \overline{AP2} | \ = | \overline{BP2} (8)
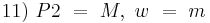\ 11) \ P2 \ = \ M, \ w \ = \ m (10, Def. Mittelpunkt, 9, Def. Mittelsenkrechte)
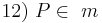\ 12) \ P \in \ m (9, 10, 11)

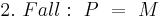
\ 2. \ Fall: \ P \ = \ M
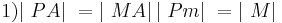\ 1) | \ PA | \ = | \ MA | \, | \ Pm | \ = | \ M | (Annahme 2. Fall)
\ 2) \ P \ ist \ Mittelpunkt \ von \overline{AB} (Def. Mittelpunkt, 1)
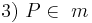\ 3) \ P \in \ m (2, Def. Mittelsenkrechte)---phil- 15:04, 5. Jul. 2011 (CEST)

Von „http://geometrie.zum.de/index.php?title=Lösung_von_Aufg._12.3_SS11&oldid=8026“