Lösung von Aufg. 10.3 S
Kopernikus / Just noch ein sailA
Beweisen Sie Satz VII.6 b
Wenn ein Punkt 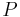 zur Mittelsenkrechten der Strecke
zur Mittelsenkrechten der Strecke  gehört, dann hat er zu den Punkten
gehört, dann hat er zu den Punkten 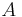 und
und 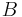 ein und denselben Abstand.
ein und denselben Abstand.
Vor:
1. 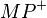 ist Mittelsenkrechte von
ist Mittelsenkrechte von 
2. 
3. 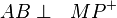
Beh:
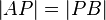
| Schritt | Beweis | Begründung |
|---|---|---|
| 1 | 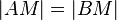
|
Vor; Def. Mittelsenkrechte. |
| 2 | 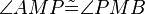
|
Axiom IV.4, Def. V.7 |
| 3 | 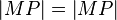
|
trivial |
| 4 | 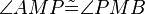
|
Axiom V, SWS |
| 5 | 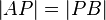
|
--Kopernikus 15:21, 28. Jun. 2012 (CEST)
--Just noch ein sailA 15:21, 28. Jun. 2012 (CEST)
Lösungsversuch Nummero6/Tchu Tcha Tcha:
Vor.: 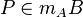
Beh.: 
(1) 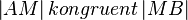 // Vor., Def. Mittelsenkrechte
// Vor., Def. Mittelsenkrechte
(2) 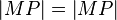 // trivial
// trivial
(3) 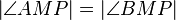 // Vor., Def. Mittelsenkrechte
// Vor., Def. Mittelsenkrechte
(4) 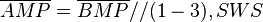
(5)  // (4), Dreieckskongruenz
// (4), Dreieckskongruenz
qed
--Tchu Tcha Tcha 12:24, 30. Jun. 2012 (CEST)
Lösungsversuch schokomuffin
Vor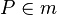 : m = Mittelsenkrechte von
: m = Mittelsenkrechte von 
Beh: 
(1) 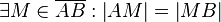 // Ex.Eind. Mittelpunkt, Ax II.2
// Ex.Eind. Mittelpunkt, Ax II.2
(2) 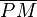 // Vor
// Vor
(3) 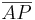 // Ax. vom Lineal
// Ax. vom Lineal
(4) 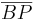 // Ax. vom Lineal
// Ax. vom Lineal
(5) 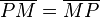 // trivial
// trivial
(6) 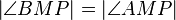 // Der. RW, NW, Vor
// Der. RW, NW, Vor
(7) 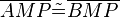 // SWS (1), (5), (6)
// SWS (1), (5), (6)
(8) 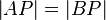 // Def. Dreieckskongurenz, (7)
// Def. Dreieckskongurenz, (7)
--schokomuffin 14:23, 01. Jul. 2012 (CEST)

