Natürliche Zahlen mit Multiplikation
Aus Geometrie-Wiki
Version vom 9. Juli 2018, 18:36 Uhr von AndyWeber (Diskussion | Beiträge)
Wir überprüfen ob es sich bei ![[\N,\sdot]](/images/math/0/3/6/0365b572ebd0ea6b7fb71b9b63d0e8da.png) um eine Gruppe handelt:
um eine Gruppe handelt:
(1) Abgeschlossenheit
Beispiel: 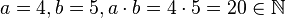 passt.
passt.
(2) Assoziativität
Beispiel: 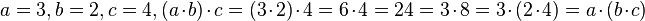 passt.
passt.
(3) neutrales Element
Beispiel: 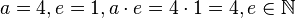 passt.
passt.
(4) inverses Element
Beispiel: 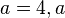 -1
-1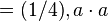 -1
-1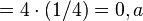 -1
-1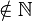 passt nicht!
passt nicht!
Somit handelt es sich bei ![[\N,\sdot]](/images/math/0/3/6/0365b572ebd0ea6b7fb71b9b63d0e8da.png) nicht um eine Gruppe.
nicht um eine Gruppe.

