Gruppen, abelsche Gruppen 2012 12: Unterschied zwischen den Versionen
Aus Geometrie-Wiki
*m.g.* (Diskussion | Beiträge) (→Restklassen modulo 4) |
*m.g.* (Diskussion | Beiträge) (→Restklassen modulo 4) |
||
| Zeile 14: | Zeile 14: | ||
#Die Verknüpfung <math>\oplus</math> ist auf der Menge <math>\mathbb{Z}_4</math> abgeschlossen, d.h. <math>\forall \overline{a}, \overline{b} \in \mathbb{Z}_4: \overline{a} \oplus \overline{b} \in \mathbb{Z}_4</math>, | #Die Verknüpfung <math>\oplus</math> ist auf der Menge <math>\mathbb{Z}_4</math> abgeschlossen, d.h. <math>\forall \overline{a}, \overline{b} \in \mathbb{Z}_4: \overline{a} \oplus \overline{b} \in \mathbb{Z}_4</math>, | ||
#Die Verknüpfung <math>\oplus</math> ist auf der Menge <math>\mathbb{Z}_4</math> assoziativ, d.h. <math>\forall \overline{a}, \overline{b}, \overline{c} \in \mathbb{Z}_4: \left(\overline{a} \oplus \overline{b} \right) \oplus \overline{c}= \overline{a} \oplus \left( \overline{b} \oplus \overline{c} \right)</math>, | #Die Verknüpfung <math>\oplus</math> ist auf der Menge <math>\mathbb{Z}_4</math> assoziativ, d.h. <math>\forall \overline{a}, \overline{b}, \overline{c} \in \mathbb{Z}_4: \left(\overline{a} \oplus \overline{b} \right) \oplus \overline{c}= \overline{a} \oplus \left( \overline{b} \oplus \overline{c} \right)</math>, | ||
| − | #<math>\mathbb{Z}_4</math> hat ein neutrales Element, nämlich die Klasse <math>\overline{0}</math>, d.h. <math> \forall \overline{a} \in \mathbb{Z}_4: \overline{a} \oplus \overline{0}= \overline{0} \oplus \overline{a} = \overline{a}</math>, | + | #<math>\mathbb{Z}_4</math> hat ein neutrales Element, nämlich die Klasse <math>\overline{0}</math>, d.h. <math> \forall \overline{a} \in \mathbb{Z}_4: \overline{a} \oplus \overline{0}= \overline{0} \oplus \overline{a} = \overline{a}</math>,<br /> |
| + | #Zu jedem Element aus <math>\mathbb{Z}_4</math> gibt es ein inverses Element, d.h. <math>\forall \overline{a} \in \mathbb{Z}_4 \exist \left(-\overline{a}\right): \overline{a} \oplus \left(-\overline{a}\right) = \left(-\overline{a}\right) \oplus \overline{a} = \overline{0} </math> | ||
Version vom 9. Dezember 2012, 18:51 Uhr
Beispiele für endliche Gruppen
Restklassen modulo 4
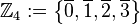
mit
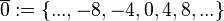(Menge aller durch 4 teilbaren ganzen Zahlen),
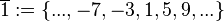(Menge aller ganzen Zahlen, die bei Division durch 4 den Rest 1 lassen),
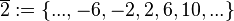(Menge aller ganzen Zahlen, die bei Division durch 4 den Rest 2 lassen),
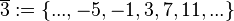(Menge aller ganzen Zahlen, die bei Division durch 4 den Rest 3 lassen),
Wir definieren auf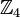 
eine Verknüpfung 
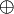wie folgt:
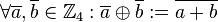
Die Struktur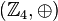 
ist eine Gruppe:
- Die Verknüpfung 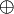ist auf der Menge 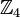abgeschlossen, d.h.
,
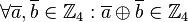- Die Verknüpfung 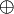ist auf der Menge 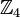assoziativ, d.h.
,
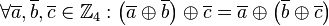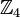- hat ein neutrales Element, nämlich die Klasse
, d.h.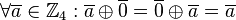 
,
- Zu jedem Element aus 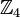gibt es ein inverses Element, d.h.
