Restklassen modulo 4 mit der Restklassenmultiplikation: Unterschied zwischen den Versionen

Aus Geometrie-Wiki
Wechseln zu: Navigation, Suche
(Die Seite wurde neu angelegt: „Wir überprüfen ob <math>[R</math><sub>4</sub><math>,\odot ]</math> eine Gruppe ist. Hierfür betrachten wir die Verknüpfungstafel: <table> <tr> <th><math>…“)
 
K
 
Zeile 16: Zeile 16:
 
<h2>Assoziativität</h2>
 
<h2>Assoziativität</h2>
  
−
<span style="color:green;">Passt</span>, denn die Restklassenmultiplikation ist definiert durch <math>\overline{a},\overline{b}\isin R</math><sub>4</sub><math>, \overline{a} \odot \overline{b} := \overline{a \sdot b}</math>
+
<span style="color:green;">Passt</span>, denn die Restklassenmultiplikation ist definiert durch <math>\overline{a},\overline{b}\isin R</math><sub>4</sub><math>, \overline{a} \odot \overline{b} := \overline{a \sdot b}</math> mit <math>a,b \isin \Z </math>
  
 
Somit lässt sich <math>(\overline{a} \odot \overline{b} )\odot \overline{c} = \overline{a} \odot (\overline{b} \odot \overline{c} )</math>
 
Somit lässt sich <math>(\overline{a} \odot \overline{b} )\odot \overline{c} = \overline{a} \odot (\overline{b} \odot \overline{c} )</math>
Zeile 22: Zeile 22:
 
auch so schreiben: <math>(\overline{a \sdot b )\sdot c} = \overline{a \sdot (b \sdot c} )</math> mit <math>a,b,c \isin \Z </math>
 
auch so schreiben: <math>(\overline{a \sdot b )\sdot c} = \overline{a \sdot (b \sdot c} )</math> mit <math>a,b,c \isin \Z </math>
  
−
und die Assoziativität in <math>\Z</math> ist bewiesen.
+
und die Assoziativität in <math>\Z</math> gilt als bewiesen.
  
 
<h2>neutrales Element</h2>
 
<h2>neutrales Element</h2>

Aktuelle Version vom 11. Juli 2018, 16:44 Uhr

Wir überprüfen ob 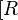[R4,\odot ] eine Gruppe ist. Hierfür betrachten wir die Verknüpfungstafel:

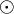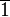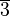\odot \overline{0}\overline{1}\overline{2}\overline{3}
\overline{0}\overline{0}\overline{0}\overline{0}\overline{0}
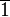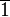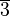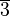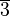\overline{1}\overline{0}\overline{1}\overline{2}\overline{3}
\overline{2}\overline{0}\overline{2}\overline{0}\overline{2}
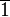\overline{3}\overline{0}\overline{3}\overline{2}\overline{1}


Inhaltsverzeichnis

Abgeschlossenheit

Passt, weil wir sehen können, dass jeder Eintrag, also jedes Ergebnis einer Restklassenmultiplikation, ebenfalls in derselben Restklasse ist.

Assoziativität

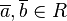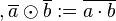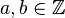
Passt, denn die Restklassenmultiplikation ist definiert durch \overline{a},\overline{b}\isin R4, \overline{a} \odot \overline{b} := \overline{a \sdot b} mit a,b \isin \Z

Somit lässt sich 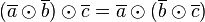(\overline{a} \odot \overline{b} )\odot \overline{c} = \overline{a} \odot (\overline{b} \odot \overline{c} )

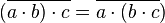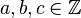
auch so schreiben: (\overline{a \sdot b )\sdot c} = \overline{a \sdot (b \sdot c} ) mit a,b,c \isin \Z

und die Assoziativität in 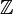\Z gilt als bewiesen.

neutrales Element

Passt, denn anhand der Verknüpfungstafel ist zu sehen, dass die 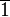\overline{1} das neutrale Element von 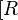[R4,\odot ] ist.

inverses Element

Passt nicht! In der Verknüpfungstafel sehen wir, das die 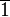\overline{1} und die 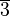\overline{3} zwar ein inverses Element haben, die \overline{2} allerdings nicht.

Resultat

Somit ist 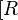[R4,\odot ] keine Gruppe.

Von „http://geometrie.zum.de/index.php?title=Restklassen_modulo_4_mit_der_Restklassenmultiplikation&oldid=32212“