Lösung von Aufgabe 8.4 (WS 11/12): Unterschied zwischen den Versionen

Aus Geometrie-Wiki
Wechseln zu: Navigation, Suche
 
(7 dazwischenliegende Versionen von 3 Benutzern werden nicht angezeigt)
Zeile 1: Zeile 1:
 
Beweisen Sie: Jede Strecke hat höchstens einen Mittelpunkt.
 
Beweisen Sie: Jede Strecke hat höchstens einen Mittelpunkt.
 
<br /><br />
 
<br /><br />
−
Vor.: Es sei AB eine Strecke und M der Mittelpunkt von AB<br /><br />
+
Vor.: Es sei <math>\overline {AB}</math> eine Strecke und M der Mittelpunkt von <math>\overline {AB}</math><br />
−
Beh.: Es existiert höchstens ein Mittelpunkt
+
Beh.: Es existiert höchstens ein Mittelpunkt<br />
−
Ann.: Es existiert M2 ein folgenden Eigenschaften: M2 ist Mittelpukt der Srecke AB und M2 ungleich M<br /><br />
+
Ann.: Es existiert <math>M_2</math> mit folgenden Eigenschaften: <math>M_2</math> ist Mittelpukt von <math>\overline {AB}</math> und <math>M_2</math> ungleich <math>M</math><br /><br />
  
 
Beweis:
 
Beweis:
Zeile 11: Zeile 11:
 
!Schritt!!Begründung
 
!Schritt!!Begründung
 
|-  
 
|-  
−
| (1)zw.(A,M,B) || Vor., Def Mittelpunkt
+
| (1)<math>Zw.(A,M,B)</math> || Vor., Def Mittelpunkt
 
|-  
 
|-  
−
| (2)zw.(A,M2,B) || Ann.
+
| (2)<math>Zw.(A,M_2,B)</math> || Ann.
 
|-
 
|-
 
| (3) <math>\left| AM \right| + \left| MB \right| = \left| AB \right|</math>    || (1),zw Relation
 
| (3) <math>\left| AM \right| + \left| MB \right| = \left| AB \right|</math>    || (1),zw Relation
 
|-
 
|-
−
| (4) <math>\left| AM \right| + \left| M2B \right| = \left| AB \right|</math>    || (2), zw Relation
+
| (4) <math>\left| AM \right| + \left| M_2B \right| = \left| AB \right|</math>    || (2), zw Relation
 
|-
 
|-
−
| (5)<math>M \neq M2</math> || Ann.
+
| (5)<math>M \neq M_2</math> || Ann.
 
|-
 
|-
−
| (6) <math>\left| AM \right| + \left| MB \right|=\left| AM \right| + \left| M2B \right|</math> || (3),(4), Rechen in R
+
| (6) <math>\left| AM \right| + \left| MB \right|=\left| AM \right| + \left| M_2B \right|</math> || (3),(4), Rechen in R
 
|-
 
|-
 
| (7) <math>\left| AM \right| = \left| MB \right|</math> || Def. Mittelpunkt (3),(4)
 
| (7) <math>\left| AM \right| = \left| MB \right|</math> || Def. Mittelpunkt (3),(4)
 
|-
 
|-
−
| (8) <math>\left| AM2 \right| = \left| M2B \right|</math> || Def.  Mittelpunkt (3),(4)
+
| (8) <math>\left| AM_2 \right| = \left| M_2B \right|</math> || Def.  Mittelpunkt (3),(4)
 
|-
 
|-
 
| (9)  <math>2\left| AM \right| = \left| AB \right|</math> || (7)
 
| (9)  <math>2\left| AM \right| = \left| AB \right|</math> || (7)
 
|-
 
|-
−
| (10) <math>2\left| AM2 \right| = \left| AB \right|</math> || (8)
+
| (10) <math>2\left| AM_2 \right| = \left| AB \right|</math> || (8)
 
|-
 
|-
−
| (11) <math>2\left| AM \right| = 2\left| M2A \right|</math> || (10)
+
| (11) <math>2\left| AM \right| = 2\left| AM_2 \right|</math> || (10)
 
|-
 
|-
−
| (12) M=M2 || (11) Axiom vom Lineal
+
| (12) <math>M=M_2</math> || (11) Axiom vom Lineal
−
|}--[[Benutzer:RicRic|RicRic]] 00:05, 6. Dez. 2011 (CET)
+
|}--[[Benutzer:RicRic|RicRic]] 00:05, 6. Dez. 2011 (CET)<br />
−
+
Nicht alle Schritte kommen in einer Begründung vor. Also brauch man diese nicht, um zu Schritt 12 zu kommen. Was meint ihr?--[[Benutzer:Tutorin Anne|Tutorin Anne]] 15:25, 7. Dez. 2011 (CET)
 +
 
 +
Also ist der Beweis richtig und ich kann Schritt (6) weglassen?--[[Benutzer:RicRic|RicRic]] 20:46, 14. Dez. 2011 (CET)ja.--[[Benutzer:Tutorin Anne|Tutorin Anne]] 16:05, 15. Dez. 2011 (CET)
  
  
  
 
[[Kategorie:Einführung_Geometrie]]
 
[[Kategorie:Einführung_Geometrie]]

Aktuelle Version vom 15. Dezember 2011, 16:05 Uhr

Beweisen Sie: Jede Strecke hat höchstens einen Mittelpunkt.

Vor.: Es sei \overline {AB} eine Strecke und M der Mittelpunkt von \overline {AB}
Beh.: Es existiert höchstens ein Mittelpunkt
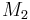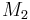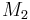Ann.: Es existiert M_2 mit folgenden Eigenschaften: M_2 ist Mittelpukt von \overline {AB} und M_2 ungleich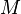 M

Beweis:


Schritt Begründung
(1)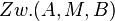Zw.(A,M,B) Vor., Def Mittelpunkt
(2)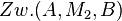Zw.(A,M_2,B) Ann.
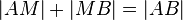(3) \left| AM \right| + \left| MB \right| = \left| AB \right| (1),zw Relation
(4) 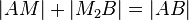\left| AM \right| + \left| M_2B \right| = \left| AB \right| (2), zw Relation
(5)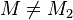M \neq M_2 Ann.
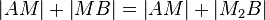(6) \left| AM \right| + \left| MB \right|=\left| AM \right| + \left| M_2B \right| (3),(4), Rechen in R
(7) \left| AM \right| = \left| MB \right| Def. Mittelpunkt (3),(4)
(8) \left| AM_2 \right| = \left| M_2B \right| Def. Mittelpunkt (3),(4)
(9) 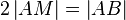2\left| AM \right| = \left| AB \right| (7)
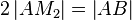(10) 2\left| AM_2 \right| = \left| AB \right| (8)
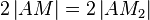(11) 2\left| AM \right| = 2\left| AM_2 \right| (10)
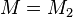(12) M=M_2 (11) Axiom vom Lineal
--RicRic 00:05, 6. Dez. 2011 (CET)

Nicht alle Schritte kommen in einer Begründung vor. Also brauch man diese nicht, um zu Schritt 12 zu kommen. Was meint ihr?--Tutorin Anne 15:25, 7. Dez. 2011 (CET)

Also ist der Beweis richtig und ich kann Schritt (6) weglassen?--RicRic 20:46, 14. Dez. 2011 (CET)ja.--Tutorin Anne 16:05, 15. Dez. 2011 (CET)

Von „http://geometrie.zum.de/index.php?title=Lösung_von_Aufgabe_8.4_(WS_11/12)&oldid=10214“