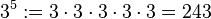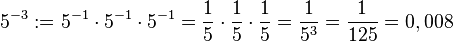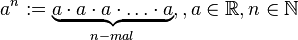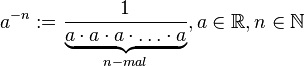Gruppenordnung, Ordnung eines Gruppenelements: Unterschied zwischen den Versionen
Aus Geometrie-Wiki
*m.g.* (Diskussion | Beiträge) |
*m.g.* (Diskussion | Beiträge) (→Potenzschreibweisen in Gruppen) |
||
| Zeile 11: | Zeile 11: | ||
=Potenzschreibweisen in Gruppen= | =Potenzschreibweisen in Gruppen= | ||
Potenzen sind aus der Schule bezüglich der Multiplikation reeller Zahlen bekannt: | Potenzen sind aus der Schule bezüglich der Multiplikation reeller Zahlen bekannt: | ||
| − | *<math> | + | *<math>3 ^5 := 3 \cdot 3 \cdot 3 \cdot 3 \cdot 3 = 243</math> |
| − | *<math>a^n := \underbrace{a \cdot a \cdot a \cdot \ldots \cdot a}_{n-mal} </math> | + | *<math>5^{-3}:=5^{-1} \cdot 5^{-1} \cdot 5^{-1} = \frac{1}{5} \cdot \frac{1}{5} \cdot \frac{1}{5}= \frac{1}{5^3}=\frac{1}{125}=0,008</math> |
| + | *<math>a^n := \underbrace{a \cdot a \cdot a \cdot \ldots \cdot a}_{n-mal}, , a \in \mathbb{R}, n \in \mathbb{N} </math> | ||
| + | *<math>a^{-n}:= \frac{1}{\underbrace{a \cdot a \cdot a \cdot \ldots \cdot a}_{n-mal}}, a \in \mathbb{R}, n \in \mathbb{N}</math> | ||
<!--- Was hier drunter steht muss stehen bleiben ---> | <!--- Was hier drunter steht muss stehen bleiben ---> | ||
|} | |} | ||
</div> | </div> | ||
[[Kategorie:Algebra]] | [[Kategorie:Algebra]] | ||
Version vom 26. November 2017, 18:10 Uhr
Die Ordnung einer GruppeDefinition (GruppenordnungEs sei BeispielePotenzschreibweisen in GruppenPotenzen sind aus der Schule bezüglich der Multiplikation reeller Zahlen bekannt: |
![[G, \odot]](/images/math/7/3/5/73557169bfbd888213d4a53d2c3ff1e4.png) eine Gruppe. Unter der Ordnung
eine Gruppe. Unter der Ordnung 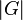 von
von 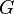 .
.
![[\mathbb{Z}_5,\oplus]: |\mathbb{Z}_5|=5](/images/math/0/a/d/0ad5cf016cd8a3a2358e7ccad3890034.png)
![[\mathbb{Z}_5,\odot]: |\mathbb{Z}_5|=4](/images/math/6/c/2/6c20f25aff626bd643056ca1a478936a.png)
![[\mathbb{Q}, +] : |\mathbb{Q}|= \infty](/images/math/6/f/2/6f2a1357b47ed4dca59f57d2db3373cf.png)