Untergruppen, Untergruppenkriterien: Unterschied zwischen den Versionen
Aus Geometrie-Wiki
*m.g.* (Diskussion | Beiträge) (→Beispiel 1) |
*m.g.* (Diskussion | Beiträge) (→Beispiel 1) |
||
| Zeile 29: | Zeile 29: | ||
<br /> | <br /> | ||
<math>[2\mathbb{Z}_6, \oplus]</math> ist eine Gruppe und damit eine Untergruppe von <math>[\mathbb{Z}_6, \oplus]</math> | <math>[2\mathbb{Z}_6, \oplus]</math> ist eine Gruppe und damit eine Untergruppe von <math>[\mathbb{Z}_6, \oplus]</math> | ||
| + | {| border="1" cellspacing="0" cellpadding="20" | ||
| + | |- | ||
| + | | <math> \oplus </math> || <math> \overline{0} </math> || <math> \overline{2} </math> || <math> \overline{4} </math> | ||
| + | |- | ||
| + | | <math> \overline{0} </math> || <math> \overline{0} </math> || <math> \overline{2} </math> || <math> \overline{4} </math> | ||
| + | |- | ||
| + | | <math> \overline{2} </math> || <math> \overline{2} </math> || <math> \overline{4} </math> || <math> \overline{0} </math> | ||
| + | |- | ||
| + | | <math> \overline{4} </math> || <math> \overline{4} </math> || <math> \overline{0} </math> || <math> \overline{2} </math> | ||
| + | |} | ||
| + | |||
| + | |||
<!--- Was hier drunter steht muss stehen bleiben ---> | <!--- Was hier drunter steht muss stehen bleiben ---> | ||
Version vom 11. Dezember 2017, 17:42 Uhr
Beispiele, GegenbeispieleBeispiel 1Wir gehen von der additiven Gruppe der Restklassen modulo 6 aus
Wir wählen aus
|
![[\mathbb{Z}_6, \oplus]](/images/math/9/0/d/90daaa2a8d1138539ff15f39a74f58c8.png) .
.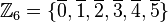
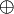

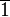

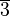
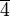
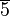
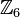 die folgende Teilmenge
die folgende Teilmenge 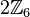 aus:
aus: 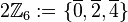
![[2\mathbb{Z}_6, \oplus]](/images/math/e/3/2/e32854e004ab70107710abac0af772d9.png) ist eine Gruppe und damit eine Untergruppe von
ist eine Gruppe und damit eine Untergruppe von 
