Lösung von Aufg. 12.6 WS 11/12: Unterschied zwischen den Versionen

Aus Geometrie-Wiki
Wechseln zu: Navigation, Suche
Zeile 10: Zeile 10:
 
   
 
   
 
   1) M sei Mittelpunkt von Strecke AB                              / Existenz und Eindeutigkeit des Mittelpunkts
 
   1) M sei Mittelpunkt von Strecke AB                              / Existenz und Eindeutigkeit des Mittelpunkts
−
   2) Es existiert C' Element MC- mit Abstand MC=MC'     / Axiom vom Lineal
+
   2) Es existiert P Element MC- mit Abstand MC=MP     / Axiom vom Lineal
 
   3) lila Winkel = lila Winkel                                              / Scheitelwinkelsatz
 
   3) lila Winkel = lila Winkel                                              / Scheitelwinkelsatz
 
   4) Strecke AM kongruent zu MB                                    / (1), Def. Mittelpunkt
 
   4) Strecke AM kongruent zu MB                                    / (1), Def. Mittelpunkt
−
   5) Strecke CM kongruent zu MC'                                   / (2)
+
   5) Strecke CM kongruent zu MP                                   / (2)
−
   6) Dreieck AMC kongruent zu Dreieck BMC                   / (3), (4), (5), SWS
+
   6) Dreieck AMC kongruent zu Dreieck BMP                   / (3), (4), (5), SWS
−
   7) <math>\  | \alpha | =\angle C'BM = 90 </math>    / (6), Vor.
+
   7) <math>\  | \alpha | =\angle PBM = 90 </math>    / (6), Vor.
 
   8) <math>\  | \beta | =  | \delta | =90 </math>          / Supplementaxiom, Vor.
 
   8) <math>\  | \beta | =  | \delta | =90 </math>          / Supplementaxiom, Vor.
−
   9) <math>\  | \delta | =\angle C'BM = 90 </math>    / (7), (8)
+
   9) <math>\  | \delta | =\angle PBM = 90 </math>    / (7), (8)
−
  10) BC- kongruent zu BC'+                                            / (9),Winkelkonstruktionsaxiom
+
  10) BC- kongruent zu BP'+                                            / (9),Winkelkonstruktionsaxiom
−
  11) C' ist Element von BC                                                / (10)
+
  11) P ist Element von BC                                                / (10)
  
−
  12) Analog lässt sich zeigen, dass C' Element von AB
+
  12) Analog lässt sich zeigen, dass P Element von AB
  
 
  13) AC kongruent zu BC                                                  / (11), (12), Axiom I.1
 
  13) AC kongruent zu BC                                                  / (11), (12), Axiom I.1

Version vom 15. Januar 2012, 14:57 Uhr

Beweisen Sie:
Korollar 1 zum schwachen Außenwinkelsatz

In jedem Dreieck sind mindestens zwei Innenwinkel spitze Winkel. 
Beweis:
Vor.: \overline{ABC} mit Fehler beim Parsen(Syntaxfehler): \  \beta   ,   \alpha    &   \gamma 

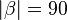
Beh.: \  | \alpha | < 90 ,  \ | \beta | < 90 
Ann.: \  | \alpha | = 90 ,  \ | \beta | = 90

Bildschirmfoto 2012-01-15 um 13.31.23.png 

 1) M sei Mittelpunkt von Strecke AB                               / Existenz und Eindeutigkeit des Mittelpunkts
 2) Es existiert P Element MC- mit Abstand MC=MP      / Axiom vom Lineal
 3) lila Winkel = lila Winkel                                              / Scheitelwinkelsatz
 4) Strecke AM kongruent zu MB                                     / (1), Def. Mittelpunkt
 5) Strecke CM kongruent zu MP                                    / (2)
 6) Dreieck AMC kongruent zu Dreieck BMP                    / (3), (4), (5), SWS
 7) \  | \alpha | =\angle PBM = 90     / (6), Vor.
 8) \  | \beta | =  | \delta | =90           / Supplementaxiom, Vor.
 9) \  | \delta | =\angle PBM = 90      / (7), (8)
10) BC- kongruent zu BP'+                                             / (9),Winkelkonstruktionsaxiom
11) P ist Element von BC                                                / (10)

12) Analog lässt sich zeigen, dass P Element von AB

13) AC kongruent zu BC                                                   / (11), (12), Axiom I.1
14) koll(ABC)                                                                    / 13), Def. koll                -> Widerspruch zur Vorrausetzung

--Flobold 13:56, 15. Jan. 2012 (CET)

Von „http://geometrie.zum.de/index.php?title=Lösung_von_Aufg._12.6_WS_11/12&oldid=10562“