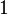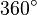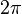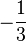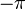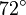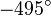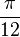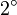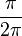Serie 5: Parameterdarstellungen I SoSe 2018: Unterschied zwischen den Versionen

Aus Geometrie-Wiki
Wechseln zu: Navigation, Suche
(→‎Aufgabe 5.1)
(→‎Aufgabe 5.1)
Zeile 15: Zeile 15:
 
| <math>1</math> || <math>360^\circ</math> || <math>2\pi</math>
 
| <math>1</math> || <math>360^\circ</math> || <math>2\pi</math>
 
|-
 
|-
−
| Beispiel || Beispiel || Beispiel
+
| <math>\frac{1}{4}</math> || <math>\ldots</math> || <math>\ldots</math>
 
|-
 
|-
−
| Beispiel || Beispiel || Beispiel
+
| <math>-\frac{1}{3}</math> || <math>\ldots</math> || <math>\ldots</math>
 
|-
 
|-
−
| Beispiel || Beispiel || Beispiel
+
| <math>\ldots</math>|| <math>\ldots</math>|| <math>-\pi</math>
 
|-
 
|-
−
| Beispiel || Beispiel || Beispiel
+
| <math>\ldots</math>|| <math>72^\circ</math>|| <math>\ldots</math>
 
|-
 
|-
−
| Beispiel || Beispiel || Beispiel
+
| <math>\frac{8}{9}</math> || <math>\ldots</math>|| <math>\ldots</math>
 
|-
 
|-
−
| Beispiel || Beispiel || Beispiel
+
| <math>\ldots</math> || <math>-495^\circ</math> || <math>\ldots</math>
 
|-
 
|-
−
| Beispiel || Beispiel || Beispiel
+
| <math>\ldots</math> || <math>\ldots</math> || <math>\frac{\pi}{12}</math>
 
|-
 
|-
−
| Beispiel || Beispiel || Beispiel
+
| <math>\ldots</math>|| <math>2^\circ</math> || <math>\ldots</math>
 
|-
 
|-
−
| Beispiel || Beispiel || Beispiel
+
| <math>\ldots</math>|| <math>\ldots</math>|| <math>\frac{\pi}{2\pi}</math>
 
|}
 
|}
 
<!--- hier drunter nichts eintragen --->
 
<!--- hier drunter nichts eintragen --->

Version vom 1. Juli 2018, 15:48 Uhr

Aufgabe 5.1

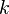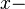Wir legen unseren Betrachtungen ein kartesisches Koordinatensystem mit dem Koordinatenursprung O zugrunde. Es seien k ein Einheitskreis in Mittelpunktslage und A der Schnittpunkt von k mit der positiven x-Achse. Der Punkt 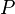P möge sich auf dem Kreis bewegen und zunächst mit $A$ zusammenfallen. Den Winkel 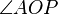\angle AOP bezeichnen wir mit \varphi. Wir verstehen \varphi als gerichteten Winkel, d.h. bewegt sich P hinreichend lange mit mathematisch negativen Drehsinn (mit dem Uhrzeigersinn) auf k wird \varphi negativ.

geogebraApp
Ergänzen Sie die folgende Tabelle:

Umdrehungen von P \varphi in Gradmaß \varphi in Bogenmaß
1 360^\circ 2\pi
\frac{1}{4} \ldots \ldots
-\frac{1}{3} \ldots \ldots
\ldots \ldots -\pi
\ldots 72^\circ \ldots
\frac{8}{9} \ldots \ldots
\ldots -495^\circ \ldots
\ldots \ldots \frac{\pi}{12}
\ldots 2^\circ \ldots
\ldots \ldots \frac{\pi}{2\pi}
Von „http://geometrie.zum.de/index.php?title=Serie_5:_Parameterdarstellungen_I_SoSe_2018&oldid=32137“