Lösung von Aufg. 7.2 (WS 11/12): Unterschied zwischen den Versionen
Aus Geometrie-Wiki
| Zeile 13: | Zeile 13: | ||
| 2) <math>\exists</math> R, Q <math>\in</math> g, R<math>\neq</math> Q || Axiom I/2 | | 2) <math>\exists</math> R, Q <math>\in</math> g, R<math>\neq</math> Q || Axiom I/2 | ||
|- | |- | ||
| − | | 3) nkoll(P, Q, R) || Axiom I/3, 1), 2) | + | | 3) nkoll(P, Q, R) || Axiom I/3, 1), 2) ''(das Axiom sagt uns nicht, dass diese drei Punkte nicht kollinear sind. Wie kann man hier anders begründen?--[[Benutzer:Tutorin Anne|Tutorin Anne]] 14:48, 29. Nov. 2011 (CET))'' |
|- | |- | ||
| 4) <math> \exists!</math> E: (P, Q, R)<math>\in</math> E || Axiom I/4, 3) | | 4) <math> \exists!</math> E: (P, Q, R)<math>\in</math> E || Axiom I/4, 3) | ||
|- | |- | ||
| − | | 5) P <math> \in</math> E <math>\wedge</math> g <math>\subseteq</math> E || 4) | + | | 5) P <math> \in</math> E <math>\wedge</math> g <math>\subseteq</math> E || 4) ''(hier noch genauer begründen --[[Benutzer:Tutorin Anne|Tutorin Anne]] 14:48, 29. Nov. 2011 (CET))'' |
|} q.e.d. --[[Benutzer:Wookie|Wookie]] 14:16, 28. Nov. 2011 (CET) | |} q.e.d. --[[Benutzer:Wookie|Wookie]] 14:16, 28. Nov. 2011 (CET) | ||
[[Category:Einführung_Geometrie]] | [[Category:Einführung_Geometrie]] | ||
Version vom 29. November 2011, 15:48 Uhr
Es sei 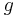 eine Gerade und
eine Gerade und 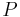 ein Punkt, der nicht zu
ein Punkt, der nicht zu 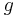 gehört. Beweisen Sie mittels der Axiome der Inzidenz: Es gibt genau eine Ebene
gehört. Beweisen Sie mittels der Axiome der Inzidenz: Es gibt genau eine Ebene  , die sowohl alle Punkte von
, die sowohl alle Punkte von 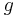 als auch den Punkt
als auch den Punkt 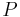 enthält.
enthält.
Voraussetzung: Gerade g, Punkt P: P 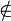 g
g
Behauptung: 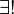 Ebene E: g
Ebene E: g 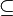 E
E 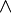 P
P 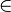 E
E
Beweis:
1) P 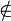 g g |
Vor. |
2)  R, Q R, Q 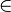 g, R g, R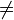 Q Q |
Axiom I/2 |
| 3) nkoll(P, Q, R) | Axiom I/3, 1), 2) (das Axiom sagt uns nicht, dass diese drei Punkte nicht kollinear sind. Wie kann man hier anders begründen?--Tutorin Anne 14:48, 29. Nov. 2011 (CET)) |
4) 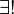 E: (P, Q, R) E: (P, Q, R)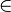 E E |
Axiom I/4, 3) |
5) P 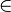 E E 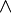 g g 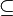 E E |
4) (hier noch genauer begründen --Tutorin Anne 14:48, 29. Nov. 2011 (CET)) |

