Lösung von Aufg. 12.7 S: Unterschied zwischen den Versionen
Aus Geometrie-Wiki
| Zeile 15: | Zeile 15: | ||
(4) <math>\gamma \tilde {=} \gamma'</math> // (1), Def. Scheitelwinkel, Scheitelwinkelsatz<br /> | (4) <math>\gamma \tilde {=} \gamma'</math> // (1), Def. Scheitelwinkel, Scheitelwinkelsatz<br /> | ||
(5) <math>\left|\alpha' \right| + \left|\beta' \right| +\left|\gamma' \right| = 180</math> // (2-4), Def.gestreckter Winkel(??)<br /> | (5) <math>\left|\alpha' \right| + \left|\beta' \right| +\left|\gamma' \right| = 180</math> // (2-4), Def.gestreckter Winkel(??)<br /> | ||
| − | (6) <math>\left|\alpha \right| + \left|\beta \right| +\left|\gamma \right| = 180</math>//(2- | + | (6) <math>\left|\alpha \right| + \left|\beta \right| +\left|\gamma \right| = 180</math>//(2-5)<br /> |
(7) Widerspruch zur Annahme // (6)<br /> | (7) Widerspruch zur Annahme // (6)<br /> | ||
(8) Behauptung stimmt // (7)<br /> | (8) Behauptung stimmt // (7)<br /> | ||
Aktuelle Version vom 13. Juli 2012, 18:52 Uhr
Beweisen Sie den Innenwinkelsatz für Dreiecke
a) mithilfe des Stufenwinkelsatzes.
b) mithilfe der Umkehrung des Stufenwinkelsatzes.
a) Lösungsversuch Nummero6/Tchu Tcha Tcha:
Vor.: Dreieck 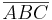 mit schulüblichen Bezeichnungen
mit schulüblichen Bezeichnungen
Beh.: 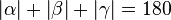
Ann.: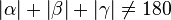
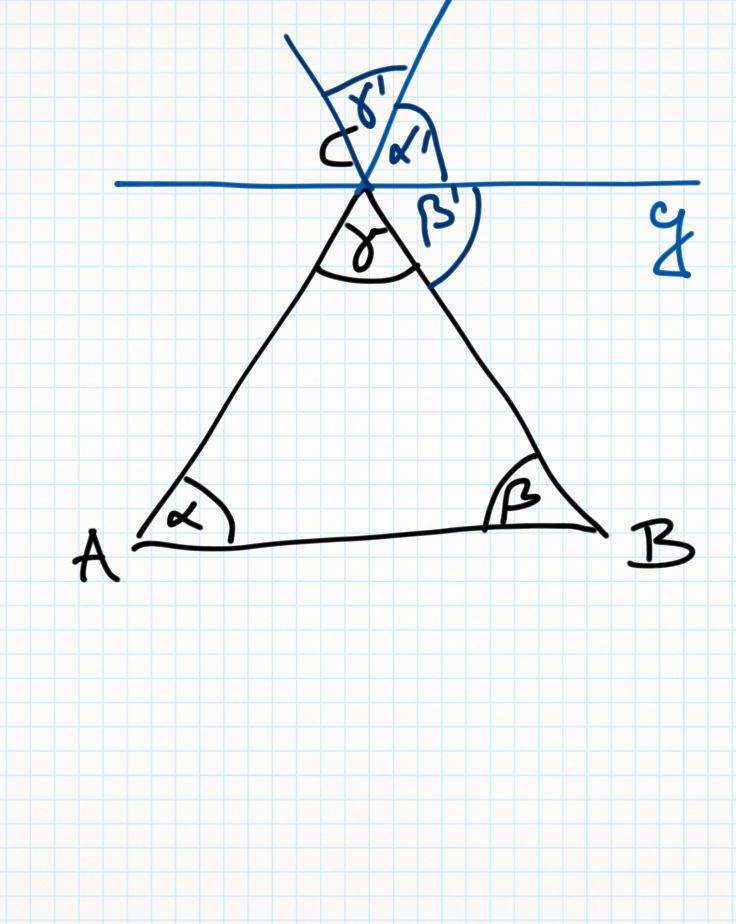
(1) 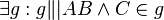 // Vor., EPA
// Vor., EPA
(2)  // (1), Def. Stufenwinkel, Stufenwinkelsatz
// (1), Def. Stufenwinkel, Stufenwinkelsatz
(3) 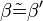 // (1), Def. Wechselwinkel, Wechselwinkelsatz
// (1), Def. Wechselwinkel, Wechselwinkelsatz
(4) 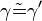 // (1), Def. Scheitelwinkel, Scheitelwinkelsatz
// (1), Def. Scheitelwinkel, Scheitelwinkelsatz
(5)  // (2-4), Def.gestreckter Winkel(??)
// (2-4), Def.gestreckter Winkel(??)
(6) 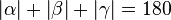 //(2-5)
//(2-5)
(7) Widerspruch zur Annahme // (6)
(8) Behauptung stimmt // (7)
qed
--Tchu Tcha Tcha 17:33, 13. Jul. 2012 (CEST)

