Übungen 08: Unterschied zwischen den Versionen
Aus Geometrie-Wiki
(Die Seite wurde neu angelegt: „=Aufgabe 1= Bestimmen Sie die Koordinaten des Vekotrs <math>\vec{x}=\begin{pmatrix} 19 \\ 5 \\ -17 \end{pmatrix}\</math> bezüglich der Basis <math>B=\{\begin{pma…“) |
(→Aufgabe 2) |
||
| Zeile 2: | Zeile 2: | ||
Bestimmen Sie die Koordinaten des Vekotrs <math>\vec{x}=\begin{pmatrix} 19 \\ 5 \\ -17 \end{pmatrix}\</math> bezüglich der Basis <math>B=\{\begin{pmatrix} 1 \\ 2 \\ 3 \end{pmatrix};\begin{pmatrix} -4 \\ -5 \\ -6 \end{pmatrix};\begin{pmatrix} 7 \\ 8 \\ 7 \end{pmatrix}\}</math> | Bestimmen Sie die Koordinaten des Vekotrs <math>\vec{x}=\begin{pmatrix} 19 \\ 5 \\ -17 \end{pmatrix}\</math> bezüglich der Basis <math>B=\{\begin{pmatrix} 1 \\ 2 \\ 3 \end{pmatrix};\begin{pmatrix} -4 \\ -5 \\ -6 \end{pmatrix};\begin{pmatrix} 7 \\ 8 \\ 7 \end{pmatrix}\}</math> | ||
| − | =Aufgabe 2= | + | ==Aufgabe 2== |
Wir betrachten in <math>\mathbb{R}^2</math> die drei Unterräume | Wir betrachten in <math>\mathbb{R}^2</math> die drei Unterräume | ||
| Zeile 16: | Zeile 16: | ||
# Es ist <math>\left\{\begin{pmatrix} 1 \\ 4 \end{pmatrix} \right\}</math> eine linear unabhängige Teilmenge von <math>U_2</math>. | # Es ist <math>\left\{\begin{pmatrix} 1 \\ 4 \end{pmatrix} \right\}</math> eine linear unabhängige Teilmenge von <math>U_2</math>. | ||
# Es gilt <math>\langle U_1 \cup U_3 \rangle = \mathbb{R}^2</math>. | # Es gilt <math>\langle U_1 \cup U_3 \rangle = \mathbb{R}^2</math>. | ||
| + | |||
| + | ==Aufgabe 3== | ||
| + | |||
| + | Überprüfen Sie, pb die folgenden 2x2-Matrizen als Linearkombinationen der Matrizen <math>\begin{pmatrix}1 & 0 \\ 1 & 1 \end{pmatrix}</math> und <math>\begin{pmatrix}1 & 1 \\ 0 & 1 \end{pmatrix}</math> darstellbar sind.<br /> | ||
| + | a) <math>\begin{pmatrix}1 & 2 \\ 3 & 4 \end{pmatrix}</math> <br /> | ||
| + | b) <math>\begin{pmatrix}5 & 3 \\ 2 & 5 \end{pmatrix}</math> | ||
| + | |||
| + | ==Aufgabe 4== | ||
| + | |||
| + | Geben Sie zu folgenden Polynomen die Koordia | ||
Version vom 26. Juni 2013, 14:14 Uhr
Inhaltsverzeichnis |
Aufgabe 1
Bestimmen Sie die Koordinaten des Vekotrs Fehler beim Parsen(Lexikalischer Fehler): \vec{x}=\begin{pmatrix} 19 \\ 5 \\ -17 \end{pmatrix}\
bezüglich der Basis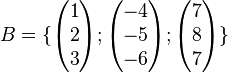
Aufgabe 2
Wir betrachten in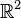 
die drei Unterräume
Fehler beim Parsen(Lexikalischer Fehler): U_1 = \left\langle \left\{\begin{pmatrix} 1 \\ 2 \end{pmatrix} \right\} \right\rangle\
,
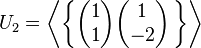und
.
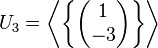Welche der folgenden Aussagen ist (sind) richtig?
- Es ist
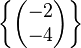ein Erzeugendensystem von 
.
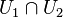- Es ist
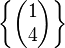eine linear unabhängige Teilmenge von 
.
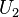- Es gilt
.
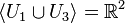Aufgabe 3
Überprüfen Sie, pb die folgenden 2x2-Matrizen als Linearkombinationen der Matrizen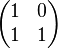 
und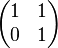 
darstellbar sind.
a)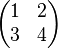
b)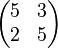
Aufgabe 4
Geben Sie zu folgenden Polynomen die Koordia
