Serie 2 Untergruppen SoSe 2017
Aus Geometrie-Wiki
Version vom 14. Mai 2017, 17:20 Uhr von *m.g.* (Diskussion | Beiträge)
Serie 2 Untergruppen SoSe 2017Aufgabe 2.1Es seien Aufgabe 2.2Es sei
Aufgabe 2.3Es sei
Aufgabe 2.4Beweisen Sie Satz 3. Aufgabe 2.5Es sei |
![[G, \odot]](/images/math/7/3/5/73557169bfbd888213d4a53d2c3ff1e4.png) und
und ![[U,\otimes]](/images/math/9/4/e/94ec02f4088cf38ce9c1c18278b7c953.png) zwei Gruppen mit
zwei Gruppen mit 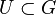 . Warum ist
. Warum ist ![[G, \otimes]](/images/math/0/1/3/01322720752789c5d9651172aee2d990.png) nach Definition 6.
nach Definition 6.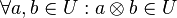 ,
,
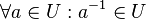 .
.
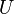 eine nichtleere Teilmenge von
eine nichtleere Teilmenge von 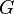 .
.![[g, \otimes]](/images/math/a/7/4/a74b84c9841f61b14965df704de3f93d.png) entsprechend Definition 6.
entsprechend Definition 6.
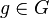 mit
mit 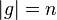 .
.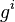 mit
mit 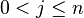 . Beweisen Sie:
. Beweisen Sie: 
