Lösung von Aufgabe 11.7: Unterschied zwischen den Versionen

Aus Geometrie-Wiki
Wechseln zu: Navigation, Suche
Zeile 51: Zeile 51:
 
|-
 
|-
 
! style="background: #FFDDDD;"|(I)
 
! style="background: #FFDDDD;"|(I)
−
| Das Dreieck <math> \overline{ABP} </math> ist ein gleichschenkliges
+
| Das Dreieck <math> \overline{ABP} </math> ist gleichschenklig
−
| (Definition gleichschenkliges Dreieck, da laut VSS <math> |AP| = |BP| </math>)
+
| Definition gleichschenkliges Dreieck, da laut VSS <math> |AP| = |BP| </math>
 
|-
 
|-
 
! style="background: #FFDDDD;"|(II)
 
! style="background: #FFDDDD;"|(II)
Zeile 63: Zeile 63:
 
|-
 
|-
 
! style="background: #FFDDDD;"|(IV)
 
! style="background: #FFDDDD;"|(IV)
−
| Die Winkelhalbierende w und die Strecke <math>\overline {AB}</math> schneiden sich in <math>S</math>, der
+
| Die Winkelhalbierende w und die Strecke <math>\overline {AB}</math> schneiden sich in <math>S</math>
 
| ... (Skizze? Reicht das als Begründung?)
 
| ... (Skizze? Reicht das als Begründung?)
 
|-
 
|-
 
! style="background: #FFDDDD;"|(VI)
 
! style="background: #FFDDDD;"|(VI)
 
| <math> \overline{APS} \cong \overline{BPS}</math>
 
| <math> \overline{APS} \cong \overline{BPS}</math>
−
| SWS: <math> \overline{AP}\cong \overline{BP}</math> (VSS) <br /> <math> \overline{PS}\cong \overline{PS}</math> (trivial) <br /><math> \delta_1 \cong \delta_2</math>  
+
| SWS: <br /><math> \overline{AP}\cong \overline{BP}</math> (VSS) <br /> <math> \overline{PS}\cong \overline{PS}</math> (trivial) <br /><math> \delta_1 \cong \delta_2</math>  
 +
|-
 +
! style="background: #FFDDDD;"|(VI)
 +
|
 +
|
 
|}
 
|}

Version vom 10. Juli 2010, 13:38 Uhr

Beweisen Sie Satz VII.6a:
Wenn ein Punkt 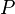P zu den Endpunkten der Strecke \overline{AB} jeweils ein und denselben Abstand hat, so ist er ein Punkt der Mittelsenkrechten von \overline{AB} .


Versuch 1:

VSS: Punkt P, \overline{AB} , |AP| = |BP| , Mittelsenkrechte m
Beh: 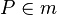P \in m

Beweis
Nr. Beweisschritt Begründung
(I) |AP| = |BP| (VSS)
(II) es existiert ein Punkt 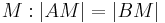M : |AM| = |BM| (Existenz und Eindeutigkeit Mittelpunkt)
(III) 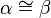\alpha \cong \beta Basiswinkelsatz
(IV) 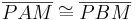\overline {PAM} \cong \overline {PBM} (I), (II), (III), (SWS)
(V) | \angle AMP| = | \angle BMP| (Def Dreieckskongruenz) (IV)
(VI) 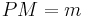PM = m (Axiom I.1), (II), (V)

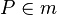--> P \in m , die Behauptung ist wahr.
qed --Löwenzahn 13:52, 4. Jul. 2010 (UTC)

Versuch 2:

VSS: Punkt P, \overline{AB} , |AP| = |BP| , Mittelsenkrechte m
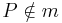
(für die gilt laut Definition: senkrecht zu \overline{AB} und geht durch M \in \overline{AB} für das gilt: |MA| = |MB| Beh: P \notin m

Beweis
Nr. Beweisschritt Begründung
(I) Das Dreieck 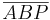\overline{ABP} ist gleichschenklig Definition gleichschenkliges Dreieck, da laut VSS |AP| = |BP|
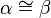
(II) \alpha \cong \beta Basiswinkelsatz
(III) Es existiert eine Winkelhalbierende w des winkels 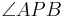\angle APB Satz VI.2 (Existenz und Eindeutigkeit der Winkelhalbierenden): Zu jedem Winkel gibt es genau eine Winkelhalbierende.
(IV) Die Winkelhalbierende w und die Strecke \overline {AB} schneiden sich in S 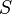... (Skizze? Reicht das als Begründung?)
(VI) 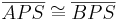\overline{APS} \cong \overline{BPS} SWS: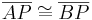
\overline{AP}\cong \overline{BP} (VSS)
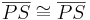\overline{PS}\cong \overline{PS} (trivial)
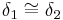
\delta_1 \cong \delta_2
(VI)
Von „http://geometrie.zum.de/index.php?title=Lösung_von_Aufgabe_11.7&oldid=2928“