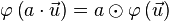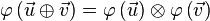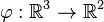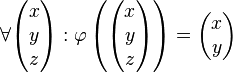Lineare Abbildungen, Vektorraumisomorphismus 2012 13: Unterschied zwischen den Versionen
Aus Geometrie-Wiki
*m.g.* (Diskussion | Beiträge) (→senkrechte Parallelprojektion) |
*m.g.* (Diskussion | Beiträge) (→senkrechte Parallelprojektion) |
||
| Zeile 7: | Zeile 7: | ||
{{Definition|(lineare Abbildung)<br />Es seien <math>\left(V_1, \oplus, \mathbb{R}, \cdot\right)</math> und <math>\left(V_2, \otimes, \mathbb{R}, \odot\right)</math> zwei Vektorräume über der Körper der reellen Zahlen. <br />Eine Abbildung <math>\varphi: V_1 \rightarrow V_2</math> heißt lineare Abbildung wenn gilt: <br /><math>\forall \vec{u}, \vec{v} \in V_1 \wedge \forall a \in \mathbb{R}:</math> <br />(H) <math>\varphi</math> ist homogen: <math>\varphi\left(a \cdot \vec{u} \right) = a \odot \varphi\left(\vec{u}\right)</math><br />(A) <math>\varphi</math> ist additiv: <math>\varphi\left(\vec{u} \oplus \vec{v} \right)= \varphi \left(\vec{u}\right) \otimes \varphi \left(\vec{v}\right)</math> }} | {{Definition|(lineare Abbildung)<br />Es seien <math>\left(V_1, \oplus, \mathbb{R}, \cdot\right)</math> und <math>\left(V_2, \otimes, \mathbb{R}, \odot\right)</math> zwei Vektorräume über der Körper der reellen Zahlen. <br />Eine Abbildung <math>\varphi: V_1 \rightarrow V_2</math> heißt lineare Abbildung wenn gilt: <br /><math>\forall \vec{u}, \vec{v} \in V_1 \wedge \forall a \in \mathbb{R}:</math> <br />(H) <math>\varphi</math> ist homogen: <math>\varphi\left(a \cdot \vec{u} \right) = a \odot \varphi\left(\vec{u}\right)</math><br />(A) <math>\varphi</math> ist additiv: <math>\varphi\left(\vec{u} \oplus \vec{v} \right)= \varphi \left(\vec{u}\right) \otimes \varphi \left(\vec{v}\right)</math> }} | ||
=Beispiele= | =Beispiele= | ||
| − | ==senkrechte Parallelprojektion== | + | ==senkrechte Parallelprojektion auf die x-y-Ebene== |
<math>\varphi: \mathbb{R}^3 \rightarrow \mathbb{R}^2</math><br /> | <math>\varphi: \mathbb{R}^3 \rightarrow \mathbb{R}^2</math><br /> | ||
<math>\forall \begin{pmatrix} x \\ y \\ z \end{pmatrix}: \varphi \left( \begin{pmatrix} x \\ y \\ z \end{pmatrix}\right)= \begin{pmatrix} x \\ y \end{pmatrix}</math><br /> | <math>\forall \begin{pmatrix} x \\ y \\ z \end{pmatrix}: \varphi \left( \begin{pmatrix} x \\ y \\ z \end{pmatrix}\right)= \begin{pmatrix} x \\ y \end{pmatrix}</math><br /> | ||
Version vom 12. Dezember 2012, 20:13 Uhr
DefinitionDefinition (lineare Abbildung) Beispielesenkrechte Parallelprojektion auf die x-y-Ebene
|
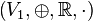 und
und 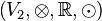 zwei Vektorräume über der Körper der reellen Zahlen.
zwei Vektorräume über der Körper der reellen Zahlen. 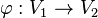 heißt lineare Abbildung wenn gilt:
heißt lineare Abbildung wenn gilt: 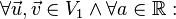
 ist homogen:
ist homogen: