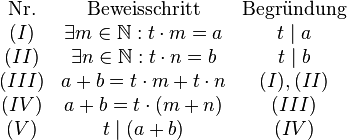Lösung von Aufgabe 2.1 SoSe 2018
Aus Geometrie-Wiki
Aufgabe 2.1 SoSe 2018Ein Blick über den Tellerrand der Geometrie: Satz S: a) Formulieren Sie Satz S schultauglich, d.h. weniger formal in einem normalen deutschen Satz. Lösunga) Wenn zwei Zahlen jeweils durch eine dritte Zahl teilbar sind, so ist auch ihre Summe durch diese dritte Zahl teilbar. |
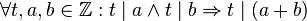
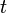 teilt sowohl
teilt sowohl  als auch
als auch 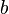 .
.