Das Lot von einem Punkt auf eine Gerade: Unterschied zwischen den Versionen
Aus Geometrie-Wiki
(→Definition IX.2: (Abstand eines Punktes zu einer Geraden)) |
(→Definition IX.2: (Abstand eines Punktes zu einer Geraden)) |
||
| (11 dazwischenliegende Versionen von 4 Benutzern werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
== Der Begriff des Lotes == | == Der Begriff des Lotes == | ||
| − | + | ||
===== Definition IX.1: (Lot, Lotgerade, Lotfußpunkt) ===== | ===== Definition IX.1: (Lot, Lotgerade, Lotfußpunkt) ===== | ||
:: Es sei <math>\ P</math> ein Punkt, der nicht zur Geraden <math>\ g</math> gehören möge. ...<br /> | :: Es sei <math>\ P</math> ein Punkt, der nicht zur Geraden <math>\ g</math> gehören möge. ...<br /> | ||
| − | :: ...Die Gerade l, die senkrecht auf g steht und durch den Punkt P geht heißt Lotgerade von P auf g. Der Schnittpunkt L von l mit g, heißt Lotfußpunkt des Lotes von P auf g. Unter dem Lot von P auf g, versteht man die Strecke <math> \overline {PL} </math>. --[[Benutzer:Löwenzahn|Löwenzahn]] 16:01, 9. Jul. 2010 (UTC) | + | :: ...Die Gerade <math>\ l</math>, die senkrecht auf <math>\ g</math> steht und durch den Punkt <math>\ P</math> geht heißt Lotgerade von <math>\ P</math> auf <math>\ g</math>. Der Schnittpunkt <math>\ L</math> von <math>\ l</math> mit <math>\ g</math>, heißt Lotfußpunkt des Lotes von <math>\ P</math> auf <math>\ g</math>. Unter dem Lot von <math>\ P</math> auf <math>\ g</math>, versteht man die Strecke <math> \overline {PL} </math>. --[[Benutzer:Löwenzahn|Löwenzahn]] 16:01, 9. Jul. 2010 (UTC) |
===== Definition IX.2: (Abstand eines Punktes zu einer Geraden) ===== | ===== Definition IX.2: (Abstand eines Punktes zu einer Geraden) ===== | ||
:: Es sei <math>\ P</math> ein Punkt außerhalb von <math>\ g</math>. Der Abstand von <math>\ P</math> zu <math>\ g</math> ist ... | :: Es sei <math>\ P</math> ein Punkt außerhalb von <math>\ g</math>. Der Abstand von <math>\ P</math> zu <math>\ g</math> ist ... | ||
| − | :: ... die Länge | + | :: ... die Länge des Lotes <math> \overline {PL} </math> von <math>\ P</math> auf <math>\ g</math>. --[[Benutzer:Löwenzahn|Löwenzahn]] 16:06, 9. Jul. 2010 (UTC) |
== Existenz und Eindeutigkeit des Lotes == | == Existenz und Eindeutigkeit des Lotes == | ||
| − | ===== Satz | + | ===== Satz IX.1: (Existenz und Eindeutigkeit des Lotes) ===== |
:: Zu jedem Punkt <math>\ P</math> außerhalb einer Geraden <math>\ g</math> gibt es genau ein Lot von <math>\ P</math> auf <math>\ g</math>. | :: Zu jedem Punkt <math>\ P</math> außerhalb einer Geraden <math>\ g</math> gibt es genau ein Lot von <math>\ P</math> auf <math>\ g</math>. | ||
| − | ===== Beweis der | + | |
| + | ===== Beweis der Existenz und Eindeutigkeit des Lotes: ===== | ||
[[Lösung von Aufgabe 12.4]] | [[Lösung von Aufgabe 12.4]] | ||
| + | |||
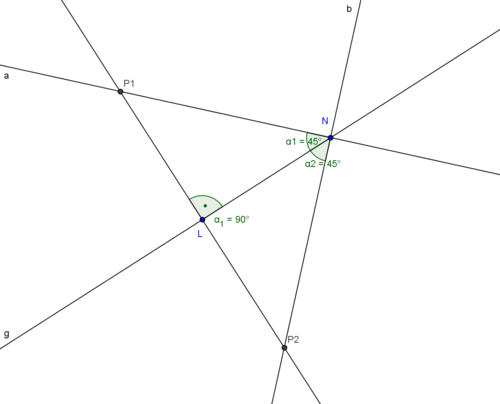
| + | [[Bild:Lot.png|500px]] | ||
| + | |||
| + | {| class="wikitable center" | ||
| + | |+ EXISTENZ | ||
| + | |- style="background: #DDFFDD;" | ||
| + | ! | ||
| + | ! Beweisschritt | ||
| + | ! Begründung | ||
| + | |- | ||
| + | ! style="background: #FFDDDD;"|(I) | ||
| + | | Konstruiere einen Punkt N auf g.<br />Fall 1: Falls <math>P1N \perp g</math>, dann ist <math>\overline{P1N}</math> unser Lot.<br />Fall 2: <math>P1N \not\perp g</math>, dann weiter mit (II) | ||
| + | | Konstruktion, (Gerade ist Menge von Punkten) | ||
| + | |- | ||
| + | ! style="background: #FFDDDD;"|(II) | ||
| + | | Antragen von <math>\alpha1: \alpha1 \cong \alpha2</math> | ||
| + | | Konstruktion, Winkelkonstruktionsaxiom | ||
| + | |- | ||
| + | ! style="background: #FFDDDD;"|(III) | ||
| + | | Antragen von <math>|NP|1: |NP1| \cong\ |NP2|</math> | ||
| + | | Konstruktion, Axiom vom Lineal | ||
| + | |- | ||
| + | ! style="background: #FFDDDD;"|(IV) | ||
| + | | Antragen von <math>|NL| \cong\ |NL|</math> | ||
| + | | trivial | ||
| + | |- | ||
| + | ! style="background: #FFDDDD;"|(V) | ||
| + | | <math>\overline{LNP1} \cong\ \overline{LNP2}</math> | ||
| + | | (II), (III), (IV), SWS | ||
| + | |- | ||
| + | ! style="background: #FFDDDD;"|(VI) | ||
| + | | <math>\angle NLP1 \cong\ \angle NLP2</math> | ||
| + | | beides rechte Winkel --> <math>\overline{PN}</math> ist Lot auf g. | ||
| + | |} | ||
| + | |||
| + | |||
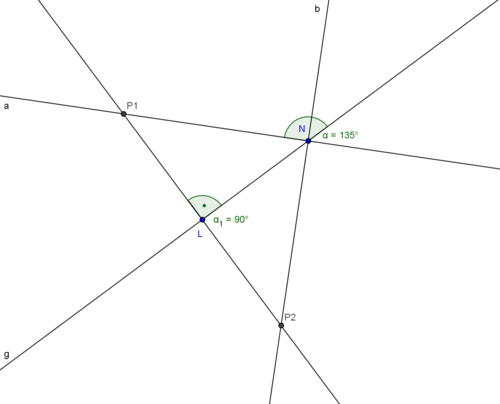
| + | [[Bild:Lot2.png|500px]] | ||
| + | |||
| + | <math> \overline{P1L} </math>ist Lot von P auf g. <br /> | ||
| + | Annahme: <math> \exists N \in g </math> mit <math>\overline{P1N} </math> ist auch Lot von P auf g, <math> L \not\equiv N.</math> <br /> <math>\alpha1 </math> ist bezüglich <math> \alpha </math> nicht anliegender Innenwinkel (<math>\overline{NLP1}</math>) --> Widerspruch, weil <math> \alpha1 < \alpha </math> (schwacher Außenwinkelsatz) | ||
Aktuelle Version vom 26. Juli 2010, 12:05 Uhr
Inhaltsverzeichnis[Verbergen] |
Der Begriff des Lotes
Definition IX.1: (Lot, Lotgerade, Lotfußpunkt)
- Es sei
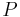 ein Punkt, der nicht zur Geraden
ein Punkt, der nicht zur Geraden 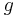 gehören möge. ...
gehören möge. ...
- ...Die Gerade
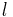 , die senkrecht auf
, die senkrecht auf 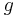 steht und durch den Punkt
steht und durch den Punkt 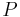 geht heißt Lotgerade von
geht heißt Lotgerade von 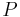 auf
auf 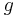 . Der Schnittpunkt
. Der Schnittpunkt  von
von 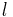 mit
mit 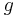 , heißt Lotfußpunkt des Lotes von
, heißt Lotfußpunkt des Lotes von 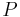 auf
auf 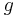 . Unter dem Lot von
. Unter dem Lot von 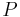 auf
auf 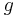 , versteht man die Strecke
, versteht man die Strecke 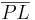 . --Löwenzahn 16:01, 9. Jul. 2010 (UTC)
. --Löwenzahn 16:01, 9. Jul. 2010 (UTC)
- Es sei
Definition IX.2: (Abstand eines Punktes zu einer Geraden)
- Es sei
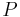 ein Punkt außerhalb von
ein Punkt außerhalb von 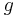 . Der Abstand von
. Der Abstand von 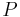 zu
zu 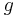 ist ...
ist ...
- ... die Länge des Lotes
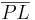 von
von 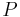 auf
auf 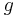 . --Löwenzahn 16:06, 9. Jul. 2010 (UTC)
. --Löwenzahn 16:06, 9. Jul. 2010 (UTC)
- Es sei
Existenz und Eindeutigkeit des Lotes
Satz IX.1: (Existenz und Eindeutigkeit des Lotes)
- Zu jedem Punkt
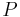 außerhalb einer Geraden
außerhalb einer Geraden 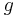 gibt es genau ein Lot von
gibt es genau ein Lot von 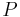 auf
auf 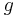 .
.
- Zu jedem Punkt
Beweis der Existenz und Eindeutigkeit des Lotes:
| Beweisschritt | Begründung | |
|---|---|---|
| (I) | Konstruiere einen Punkt N auf g. Fall 1: Falls 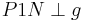 , dann ist , dann ist 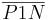 unser Lot. unser Lot.Fall 2: 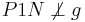 , dann weiter mit (II) , dann weiter mit (II)
|
Konstruktion, (Gerade ist Menge von Punkten) |
| (II) | Antragen von 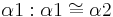
|
Konstruktion, Winkelkonstruktionsaxiom |
| (III) | Antragen von 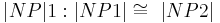
|
Konstruktion, Axiom vom Lineal |
| (IV) | Antragen von 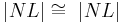
|
trivial |
| (V) | 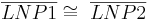
|
(II), (III), (IV), SWS |
| (VI) | 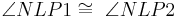
|
beides rechte Winkel -->  ist Lot auf g. ist Lot auf g.
|
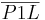 ist Lot von P auf g.
ist Lot von P auf g.
Annahme: 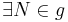 mit
mit 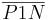 ist auch Lot von P auf g,
ist auch Lot von P auf g, 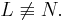
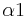 ist bezüglich
ist bezüglich 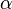 nicht anliegender Innenwinkel (
nicht anliegender Innenwinkel ( ) --> Widerspruch, weil
) --> Widerspruch, weil 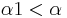 (schwacher Außenwinkelsatz)
(schwacher Außenwinkelsatz)