Lösung von Aufg. 13.2 (WS 11/12): Unterschied zwischen den Versionen
Aus Geometrie-Wiki
RicRic (Diskussion | Beiträge) |
Adores (Diskussion | Beiträge) (table+ table+ table+) |
||
| Zeile 2: | Zeile 2: | ||
Kann man, um diese Implikation zu beweisen, das Parallelnaxiom verwenden? | Kann man, um diese Implikation zu beweisen, das Parallelnaxiom verwenden? | ||
| − | * Mann kann es in der absoluten Geometrie beweisen. D.h. ohne Parallelaxiom. --[[Benutzer:RicRic|RicRic]] 07:53, 23. Jan. 2012 (CET) | + | * Mann kann es in der absoluten Geometrie beweisen. D.h. ohne Parallelaxiom. --[[Benutzer:RicRic|RicRic]] 07:53, 23. Jan. 2012 (CET |
| + | |||
| + | <br /> | ||
| + | Vor: P, g, P <math>\not\in</math> g <br /> | ||
| + | Beh: P <math>\in</math> h <math>\wedge</math> <math>h\|| g</math> | ||
| + | |||
| + | |||
| + | {| class="wikitable " | ||
| + | ! Beweisschritt | ||
| + | ! Begründung | ||
| + | |- | ||
| + | | 1) <math>\exists</math> R, L : R,L <math>\in</math> g | ||
| + | | Axiom I.2 | ||
| + | |- | ||
| + | | 2) <math>\exists</math> l: P, L <math>\in</math> l <math>\wedge</math> <math>\ l \perp \ g</math> <math>\wedge</math> <math>\ l \cap g</math> = {L} | ||
| + | | Ex. und Eind. Lot, (1) | ||
| + | |- | ||
| + | | 3) <math>\exists</math> Q: Q <math>\in</math> gP<sup>+</sup> <math>\wedge</math> <math>Q \neq P</math> | ||
| + | | Definition Halbebene | ||
| + | |- | ||
| + | | 4) <math>\exists</math> PA<sup>+</sup>: <math>\angle APL</math> = 90 <math>\wedge</math> PA<sup>+</sup> Teilmenge von lQ<sup>+</sup> | ||
| + | | Axiom IV.2, (2), (3) | ||
| + | |- | ||
| + | | 5) <math>\angle APL</math> <math>\tilde {=}</math> <math>\angle RLP</math> | ||
| + | |(2), (4) | ||
| + | |- | ||
| + | | 6) <math>h\|| g</math> | ||
| + | | (5), Umkehrung Wechselwinkelsatz | ||
| + | |- | ||
| + | | q.e.d. | ||
| + | | | ||
| + | |} | ||
| + | --[[Benutzer:Adores|Adores]] 01:36, 24. Jan. 2012 (CET) | ||
| + | |||
| + | |||
[[Category:Einführung_Geometrie]] | [[Category:Einführung_Geometrie]] | ||
Version vom 24. Januar 2012, 02:36 Uhr
Beweisen Sie: Wenn 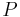 ein Punkt außerhalb der Geraden
ein Punkt außerhalb der Geraden 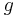 ist, dann gibt es eine Gerade
ist, dann gibt es eine Gerade 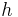 , die durch
, die durch 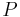 geht und parellel zu
geht und parellel zu 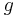 ist.
ist.
Kann man, um diese Implikation zu beweisen, das Parallelnaxiom verwenden?
- Mann kann es in der absoluten Geometrie beweisen. D.h. ohne Parallelaxiom. --RicRic 07:53, 23. Jan. 2012 (CET
Vor: P, g, P 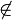 g
g
Beh: P 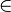 h
h 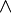
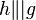
| Beweisschritt | Begründung |
|---|---|
1)  R, L : R,L R, L : R,L 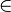 g g
|
Axiom I.2 |
2)  l: P, L l: P, L 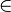 l l 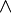 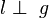 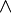 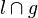 = {L} = {L}
|
Ex. und Eind. Lot, (1) |
3)  Q: Q Q: Q 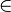 gP+ gP+ 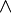 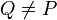
|
Definition Halbebene |
4)  PA+: PA+: 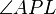 = 90 = 90 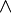 PA+ Teilmenge von lQ+ PA+ Teilmenge von lQ+
|
Axiom IV.2, (2), (3) |
5) 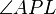 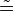 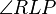
|
(2), (4) |
6) 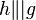
|
(5), Umkehrung Wechselwinkelsatz |
| q.e.d. |
--Adores 01:36, 24. Jan. 2012 (CET)

